VaR Mapping - FRM Part 2
Learning Objectives
- Explain the principles underlying VaR mapping, and describe the mapping process.
- Explain how the mapping process captures general and specific risks.
- Differentiate among the three methods of mapping portfolios of fixed income securities.
- Summarize how to map a fixed income portfolio into positions of standard instruments.
- Describe how mapping of risk factors can support stress testing.
- Explain how VaR can be used as a performance benchmark.
- Video Lecture
- |
- PDFs
- |
- List of chapters
Chapter Contents
- Three Approaches For Fixed Income Portfolios
- Mapping Fixed Income Portfolios
- Mapping Fixed Income Portfolios-CF Mapping
- Three Approaches For Fixed Income Portfolios
- Stress Testing
- Benchmarking
- Mapping Forwards
- Mapping Commodity Forwards
- Mapping Forward Rate Agreements
- Mapping Interest Rate Swap
- Mapping Options
- General and Specific Risks
- Three Approaches For Fixed Income Portfolios
- Mapping Fixed Income Portfolios
- Mapping Fixed Income Portfolios-CF Mapping
- Three Approaches For Fixed Income Portfolios
- Stress Testing
- Benchmarking
- Mapping Forwards
- Mapping Commodity Forwards
- Mapping Forward Rate Agreements
- Mapping Interest Rate Swap
- Mapping Options
- General and Specific Risks
- Three Approaches For Fixed Income Portfolios
- Mapping Fixed Income Portfolios
- Mapping Fixed Income Portfolios-CF Mapping
- Three Approaches For Fixed Income Portfolios
- Stress Testing
- Benchmarking
- Mapping Forwards
- Mapping Commodity Forwards
- Mapping Forward Rate Agreements
- Mapping Interest Rate Swap
- Mapping Options
- Principle Underlying VaR Mapping
- Advantages of VaR Mapping
- Disadvantages of VaR Mapping
- General Mapping Process
- General and Specific Risks
- Three Approaches For Fixed Income Portfolios
- Mapping Fixed Income Portfolios
- Mapping Fixed Income Portfolios-CF Mapping
- Three Approaches For Fixed Income Portfolios
- Stress Testing
- Benchmarking
- Mapping Forwards
- Mapping Commodity Forwards
- Mapping Forward Rate Agreements
- Mapping Interest Rate Swap
- Mapping Options
- The choice of primitive risk factors also influences the size of specific risks. Specific risk can be defined as risk that is due to issuer-specific price movements, after accounting for general market factors.
- The Basel rules have a separate charge for specific risk. Typically, the charge is 4 percent of the position value for equities and unrated debt, assuming that the banks’ models do not incorporate specific risks.
- To illustrate this decomposition, consider a portfolio of 𝑁 stocks. We are mapping each stock on a position in the stock market index, which is our primitive risk factor. The return on a stock 𝑅i is regressed on the return on the stock market index 𝑅m, i.e.,
α, which does not contribute to risk, is ignored. We assume that the specific risk owing to 𝜖 is not correlated across stocks or with the market. The relative weight of each stock in the portfolio is given by 𝑤i. Thus the portfolio return is
where 𝛽P = Beta of the portfolio, which is the weighted average of the individual betas
Now, let’s decompose the variance of the portfolio return
The first component is the general market risk. The second component is the aggregate of specific risk for the entire portfolio. This decomposition shows that with more detail on the primitive or general-market risk factors, there will be less specific risk for a fixed amount of total risk 𝑉𝑎𝑟(𝑅p).
So if there are two identical portfolios of stocks, where one is mapped to only one general risk factor, and the other is mapped to many general risk factors, then the specific risk for the second portfolio will be lesser.
- We have to restrict the number of risk factors to a small set. For some portfolios, one risk factor may be sufficient. For others, many may be necessary. For option portfolios, we need to model movements in their implied volatilities as well, apart from yields
- For a portfolio of bonds, the primitive risk factors could be movements in a set of 𝐽 government bond yields 𝑧j and in a set of 𝐾 credit spreads 𝑠k sorted by credit rating. We model the movement in each corporate bond yield 𝑑𝑦i, by a movement in z at the closest maturity and in 𝑠 for the same credit rating. The remaining component is si
The movement in the value, say 𝑊 then is
where 𝐷𝑉𝐵𝑃 is the total dollar value of a basis point for the associated risk factor.
This leads to a total risk decomposition of
A greater number of general risk factors should create less residual risk. In practice, there may not be sufficient history to measure the specific risk of individual bonds, which is why i is often assumed that all issuers within the same risk class have the same risk.
Three Approaches For Fixed Income Portfolios
- The three approaches to mapping portfolios of fixed income securities are:
- Principal mapping – One risk factor is chosen that corresponds to the average portfolio maturity. Coupon is stripped out and only principal repayment is considered at maturity.
- Duration mapping – One risk factor is chosen that corresponds to the portfolio duration. Coupons are incorporated along with the principal.
- Cash flow mapping – Several risk factor are chosen and the bond risk is decomposed into risk of each of the bond cash flows. The portfolio cash flows are grouped into maturity buckets. Cash flow mapping is the most precise method because present values of the cash flows are considered, and inter-maturity correlations are included.
Mapping Fixed Income Portfolios
| Term | Yield | Yield VaR | Modified Duration | Returns VaR |
|---|---|---|---|---|
| 1 | 5.830% | 0.00497401 | 0.944911651 | 0.470% |
| 2 | 5.710% | 0.005216789 | 1.891968593 | 0.987% |
| 3 | 5.810% | 0.005234068 | 2.835270768 | 1.484% |
| 4 | 5.890% | 0.00521773 | 3.777504958 | 1.971% |
| 5 | 5.960% | 0.005141179 | 4.718761797 | 2.426% |
| 7 | 6.070% | 0.004836792 | 6.59941548 | 3.192% |
| 9 | 6.200% | 0.00462442 | 8.474576271 | 3.919% |
| 10 | 6.260% | 0.00451605 | 9.410878976 | 4.250% |
| Term (Year) | Cash Flows | Spot Rate | Mapping (PV) | |||
|---|---|---|---|---|---|---|
| 5 Year Bond | 1 Year Bond | Principal | Duration | Cash Flow | ||
| 1 | $6 | $104 | 4.000% | 0 | 0 | $105.77 |
| 2 | $6 | 0 | 4.618% | 0 | 0 | $5.48 |
| 2.733 | — | — | — | — | $200 | — |
| 3 | $6 | 0 | 5.192% | $200 | 0 | $5.15 |
| 4 | $6 | 0 | 5.716% | 0 | 0 | $4.80 |
| 5 | $106 | 0 | 6.112% | 0 | 0 | $78.79 |
| Total | — | — | $200 | $200 | $200 | |
Mapping Fixed Income Portfolios-CF Mapping
| Term (Year) | PV Cash Flows | Individual VaR | Correlation Matrix R | Component VaR | |||||
|---|---|---|---|---|---|---|---|---|---|
| x | x × V | 1Y | 2Y | 3Y | 4Y | 5Y | (xΔVaR) | ||
| 1 | $105.77 | 0.4966 | 1 | – | – | – | – | $0.45 | |
| 2 | $5.48 | 0.0540 | 0.897 | 1 | – | – | – | $0.05 | |
| 3 | $5.15 | 0.0765 | 0.886 | 0.991 | 1 | – | – | $0.08 | |
| 4 | $4.80 | 0.0947 | 0.866 | 0.976 | 0.994 | 1 | – | $0.09 | |
| 5 | $78.79 | 1.9115 | 0.855 | 0.966 | 0.988 | 0.998 | 1 | $1.90 | |
| Total | $200.00 | 2.6335 | |||||||
| Undiversified VaR | $2.63 | ||||||||
| Diversified VaR | $2.57 | ||||||||
Three Approaches For Fixed Income Portfolios
- Cash Flow mapping VaR is less than the duration VaR because of two reasons :
- First, risk measures are not perfectly linear with maturity
- Second, correlations are below unity, which reduces risk even further.
- Out of the total $130,000 difference ($2.70 million – $2.57 million) in these measures, $70,000 ($2.70-$2.63 million) is due to differences in yield volatility, and the remaining $60,000 is due to imperfect correlations.
Stress Testing
COMPUTING VAR FROM CHANGE IN PRICE OF ZEROS
| Term (Year) | Cash Flows | Spot Rate | Old Discount Factor | PV Of CF | Risk (%) | New Discount Factor | New PV of CF |
|---|---|---|---|---|---|---|---|
| 1 | $110 | 4.000% | 0.96154 | $105.769 | 0.470% | 0.95702 | $105.272 |
| 2 | $6 | 4.618% | 0.91367 | $5.482 | 0.987% | 0.90465 | $5.428 |
| 3 | $6 | 5.192% | 0.85912 | $5.155 | 1.484% | 0.84637 | $5.078 |
| 4 | $6 | 5.716% | 0.80064 | $4.804 | 1.971% | 0.78486 | $4.709 |
| 5 | $106 | 6.112% | 0.74332 | $78.792 | 2.426% | 0.72529 | $76.881 |
| Total | $200.00 | $197.368 | |||||
| Loss | $2.634 | ||||||
Benchmarking
| Vertex | Risk (%) | Position: Index ($) | Position: Portfolio | ||||
|---|---|---|---|---|---|---|---|
| 1 ($) | 2 ($) | 3 ($) | 4 ($) | 5 ($) | |||
| ≤1m | 0.022 | 1.05 | 0.0 | 0.0 | 0.0 | 0.0 | 84.8 |
| 3m | 0.065 | 1.35 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 6m | 0.163 | 2.49 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 1Y | 0.470 | 13.96 | 0.0 | 0.0 | 59.8 | 0.0 | 0.0 |
| 2Y | 0.987 | 24.83 | 0.0 | 62.6 | 0.0 | 0.0 | 0.0 |
| 3Y | 1.484 | 15.40 | 0.0 | 59.5 | 0.0 | 0.0 | 0.0 |
| 4Y | 1.971 | 11.57 | 38.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 5Y | 2.426 | 7.62 | 62.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 7Y | 3.192 | 6.43 | 0.0 | 40.5 | 0.0 | 0.0 | 0.0 |
| 9Y | 3.913 | 4.51 | 0.0 | 37.4 | 0.0 | 0.0 | 0.0 |
| 10Y | 4.250 | 3.34 | 0.0 | 0.0 | 40.2 | 0.0 | 0.0 |
| 15Y | 6.234 | 3.00 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 20Y | 8.146 | 3.15 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 30Y | 11.119 | 1.31 | 0.0 | 0.0 | 0.0 | 0.0 | 15.2 |
| Total | 100.00 | 100.00 | |||||
| Duration | 4.62 | ||||||
| Absolute VaR | $1.99 $2.25 $2.16 $2.04 $1.94 $1.71 | ||||||
| Tracking error VaR | $0.00 $0.43 $0.29 $0.16 $0.20 $0.81 | ||||||
This table presents the cash-flow decomposition of the J.P. Morgan U.S. bond index, which has a duration of 4.62 years. Assume that we are trying to benchmark a portfolio of $100 million. Over a monthly horizon, the VaR of the index at the 95 percent confidence level is $1.99 million. This is about equivalent to the risk of a 4-year note.
- We try to match the index with two bonds. The rightmost columns in the table display the positions of two-bond portfolios with duration matched to that of the index. Since no zero- coupon has a maturity of exactly 4.62 years, the closest portfolio consists of two positions, each in a 4- and a 5-year zero. The respective weights for this portfolio are $38 million and $62 million.
If we define the new vector of positions for this portfolio as 𝑥 and for the index as 𝑥0. The VaR of the deviation relative to the benchmark is
Mapping Forwards
- From Part 1, we know that if we assume continuous compounding, then the current value of the forward contract is
And for discrete compounding it can be written as
For currency forwards, the foreign interest rate will take the place of 𝑦 in the above equations.
Apart from that it can also be expressed as the is the present value of the difference between the current forward rate and the locked-in delivery rate, i.e.
So, if we are long a forward contract with contracted rate 𝐾, we can liquidate the contract by entering a new contract to sell at the current rate 𝐹t. This will lock in a profit of (𝐹t – 𝐾), which we need to discount to the present time to find 𝐹t .
Risk and Correlations for Forward Contract Risk Factors (monthly VaR at 95 percent level)
| Risk Factor | Price or Rate | VaR (%) | Correlations | ||
|---|---|---|---|---|---|
| EUR Spot | EUR 1Y | USD 1Y | |||
| EUR spot | $1.2877 | 0.0400 | |||
| Long EUR bill | 2.2810% | -0.0583 | |||
| Short EUR bill | 3.3304% | 0.2121 | 0.0400 | -0.0583 | 1 |
| EUR forward | $1.3009 | ||||
- This table examines the risk of a 1-year forward contract to purchase 100 million euros in exchange for $130.086 million. The first step is to find the market value of the contract using the previous equation for discrete compounding
Thus the initial value of the contract is zero. This value may change, creating market risk.
- Among the three sources of risk, the volatility of the spot contract is the highest by far, with a 4.54 percent VaR (corresponding to 1.65 standard deviations over a month for a 95 percent confidence level). This is much greater than the 0.14 percent VaR for the EUR 1-year bill or even the 0.21 percent VaR for the USD bill. Thus most of the risk of the forward contract is driven by the cash EUR position.
- But risk is also affected by correlations. The positive correlation of 0.13 between the EUR spot and bill positions indicates that when the EUR goes up in value against the dollar, the value of a 1-year EUR investment is likely to appreciate. Therefore, higher values of the EUR are associated with lower EUR interest rates. This positive correlation increases the risk of the combined position. On the other hand, the position is also short a 1-year USD bill, which is correlated with the other two legs of the transaction. The issue is, what will be the net effect on the risk of the forward contract?
Computing VaR for a EUR 100 Million Forward Contract (monthly VaR at 95 percent level)
| Position | Present-value Factor | Cash Flow (CF) | PV of Flows, \(x\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| EUR spot | — | $125.89 | $5.713 | $5.704 | |
| Long EUR bill | 0.977698 | EUR100.00 | $125.89 | $0.176 | $0.029 |
| Short EUR bill | 0.967769 | —130.09 | —125.89 | $0.267 | $0.002 |
| Undiversified VaR | — | $6.156 | — | ||
| Diversified VaR | — | — | $5.735 | ||
- A long position in a forward contract that is used to purchase foreign currency with USD, one year from now, has three components:
- Long position in foreign currency spot i.e. a long spot position in EUR, worth EUR 100 million = $130.09 million in a year, or ((𝑆𝑒–rc) = $125.89 million now
- Long position in foreign currency bill i.e. a long position in a EUR investment, also worth $125.89 million now,
- Short position in USD bill i.e. a short position in a USD investment, worth $130.09 million i a year, or (𝐾𝑒–rc) = $125.89 million now.
- Considering only the spot position, the VaR is $125.89 million times the risk of 4.538 percent, which is $5.713 million. To compute the diversified VaR, we use the risk matrix from the data in the previous table and pre- and post-multiply by the vector of positions (PV of flows column in the table). The total VaR for the forward contract is $5.735 million. This number is about the same size as that of the spot contract because exchange-rate volatility dominates the volatility of 1-year bonds.
- More generally, the same methodology can be used for long-term currency swaps, which are equivalent to portfolios of forward contracts. For instance, a 10-year contract to pay dollars and receive euros is equivalent to a series of 10 forward contracts to exchange a set amount of dollars into marks. To compute the VaR, the contract must be broken down into a currency-risk component and a string of USD and EUR fixed-income components. As before, the total VaR will be driven primarily by the currency component.
Mapping Commodity Forwards
- The valuation of forward or futures contracts on commodities is substantially more complex than for financial assets such as currencies, bonds, or stock indices. Most commodities do not make monetary payments but instead are consumed, thus creating an implied benefit. This flow of benefit, net of storage cost, is loosely called convenience yield to represent the benefit from holding the cash product. This convenience yield, however, is not tied to another financial variable, such as the foreign interest rate for currency futures. It is also highly variable, creating its own source of risk.
- Monthly VaR measures for commodity futures are very high, in contrast to currency and equity market VaRs. Thus commodities are much more volatile than typical financial assets.
- Volatilities decrease with maturity of commodity futures contracts. The effect is strongest for less storable products such as energy products and less so for base metals. And this effect is very light for precious metals, which have low storage costs and no convenience yield. For financial assets, volatilities are driven primarily by spot prices, which implies basically constant volatilities across contract maturities.
- The forward rate agreement can be decomposed into two zero coupon components. If long position in a 6 x 12 FRA is considered, the components are:
- Long position in a 6-month bill
- Short position in a 12-month bill
Computing the VaR of a $100 FRA (monthly VaR at 95 percent level)
| Position | PV of Flows \(x\) | Risk % \(V\) | Correlation Matrix \(R\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| 180 days | —$97.264 | 0.1629 | 1 0.8738 |
$0.158 | $5.704 |
| 360 days | $97.264 | 0.4696 | 0.8738 1 |
$0.457 | $0.029 |
| Undiversified VaR | — | $0.615 | — | ||
| Diversified VaR | — | — | $0.327 | ||
his table provides a worked-out example. If the 360-day spot rate is 5.8125 percent and the 180-day rate is 5.6250 percent, the forward rate must be such that
The present value of the notional $100 million in 6 months is
This amount is invested for 12 months.
Mapping Forward Rate Agreements
- The previous table also displays the computation of VaR for the FRA. The VaRs of 6- and 12-month zeroes are 0.1629 and 0.4696, respectively, with a correlation of 0.8738. Applied to the principal of $97.26 million, the individual VaRs are $0.158 million and $0.457 million, which gives an undiversified VaR of $0.615 million. Fortunately, the correlation substantially lowers the FRA risk. The largest amount the position can lose over a month at the 95 percent level is $0.327 million.
Mapping Interest Rate Swap
- Interest-rate swaps are the most actively used derivatives. They create exchanges of interest- rate flows from fixed to floating or vice versa. Swaps can be decomposed into two legs, a fixed leg and a floating leg. The fixed leg can be priced as a coupon-paying bond; the floating leg is equivalent to a floating-rate note (FRN).
- To illustrate, let us compute the VaR of a $100 million 5-year interest-rate swap. We enter a dollar swap that pays 6.195 percent annually for 5 years in exchange for floating-rate payments indexed to London Interbank Offer Rate (LIBOR). Initially, we consider a situation where the floating-rate note is about to be reset. Just before the reset period, we know that the coupon will be set at the prevailing market rate. Therefore, the note carries no market risk, and its value can be mapped on cash only. Right after the reset, however, the note becomes similar to a bill with maturity equal to the next reset period.
- Interest-rate swaps can be viewed in two different ways: as (1) a combined position in a fixed- rate bond and in a floating-rate bond or (2) a portfolio of forward contracts. We first value the swap as a position in two bonds using risk VaR data from the earlier table that we used to map fixed income securities. The analysis is detailed in table given in the next slide.
Computing the VaR of a $100 million Interest Rate Swap (monthly VaR at 95 percent level)
| Term (Year) | Cash Flow | Spot Rate | PV of Net Cash Flow | Individual VaR | Component VaR | |
|---|---|---|---|---|---|---|
| Fixed | Floating | |||||
| 0 | $0 | +100 | —$100.000 | $0 | $0 | |
| 1 | —$6.195 | $0 | 5.813% | —$5.855 | $0.027 | $0.024 |
| 2 | —$6.195 | $0 | 5.929% | —$5.521 | $0.054 | $0.053 |
| 3 | —$6.195 | $0 | 6.034% | —$5.196 | $0.077 | $0.075 |
| 4 | —$6.195 | $0 | 6.130% | —$4.833 | $0.096 | $0.096 |
| 5 | —$106.195 | $0 | 6.217% | —$78.546 | $1.905 | $1.905 |
| Total | $0.000 | |||||
| Undiversified VaR | $2.160 | |||||
| Diversified VaR | $2.152 | |||||
- The second and third columns lay out the payments on both legs. Just before reset, the long position in the FRN is worth $100 million, and the market value of the swap is zero. To clarify the allocation of current values, the FRN is allocated to cash, with a zero maturity. This has no risk. The next column lists the zero-coupon swap rates for maturities going from 1 to 5 years. The fifth column reports the present value of the net cash flows, fixed minus floating. The last column presents the component VaR, which adds up to a total diversified VaR of $2.152 million. The undiversified VaR is obtained from summing all individual VaRs. As usual, the $2.160 millio value somewhat overestimates risk.
- This swap can also be viewed as the sum of five forward contracts, as shown in this table. The 1-year contract promises payment of $100 million plus the coupon of 6.195 percent; discounted at the spot rate of 5.813 percent, this yields a present value of -$100.36 million. This is in exchange for $100 million now, which has no risk.
An Interest-Rate Swap Viewed as Forward Contracts (monthly VaR at 95 percent level)
| Term (Year) | PV of Flows: Contract | VaR | ||||
|---|---|---|---|---|---|---|
| 1 | 1 × 2 | 2 × 3 | 3 × 4 | 4 × 5 | ||
| 1 | —$100.36 | $94.50 | ||||
| 2 | -$94.64 | $89.11 | ||||
| 3 | $89.08 | $83.88 | ||||
| 4 | -$83.70 | $78.82 | ||||
| 5 | —$78.55 | |||||
| VaR | $0.471 | $0.571 | $0.488 | $0.446 | $0.425 | |
| Undiversified VaR | $2.401 | |||||
| Diversified VaR | $2.152 | |||||
- The next contract is a 1 x 2 forward contract that promises to pay the principal plus the fixed coupon in 2 years, or -$106.195 million; discounted at the 2-year spot rate, this yields -$94.64 million. This is in exchange for $100 million in 1 year, which is also $94.50 million when discounted at the 1-year spot rate. And so on until the fifth contract, a 4 x 5 forward contract.
- The previous table also shows the VaR of each contract. The undiversified VaR of $2.401 million is the result of a simple summation of the five VaRs. The fully diversified VaR is
- $2.152 million, exactly the same as in the preceding table. This demonstrates the equivalence of the two approaches.
- Finally, we examine the change in risk after the first payment has just been set on the floating- rate leg. The FRN then becomes a 1-year bond initially valued at par but subject to fluctuations in rates. The only change in the pattern of cash flows in Table 4-10 is to add $100 million to the position on year 1 (from -$5.855 to $94.145). The resulting VaR then decreases from $2.152 million to $1.763 million. More generally, the swap’s VaR will converge to zero as the swap matures, dipping each time a coupon is set.
Mapping Options
- Let’s consider the mapping process for nonlinear derivatives, or options. Obviously, this nonlinearity may create problems for risk measurement systems based on the delta-normal approach, which is fundamentally linear. To simplify, consider the Black-Scholes (BS) model for European options. From Part 1, we remember that
where 𝑁(𝑑) is the cumulative normal distribution function with arguments, and
where 𝐾 is now the exercise price at which the option holder can, but is not obligated to, buy the asset.
- Delta, is particularly important for options. For a European call, this is
. This figure displays its behavior as a function of the underlying spot price and for various maturities. The figure shows that delta is not a constant, which may make linear methods inappropriate for measuring the risk of options. Delta increases with the underlying spot price. The relationship becomes more nonlinear for short-term options, for example, with an option maturity of 10 days. Linear methods approximate delta by a constant value over the risk horizon. The quality of this approximation depends on parameter values.
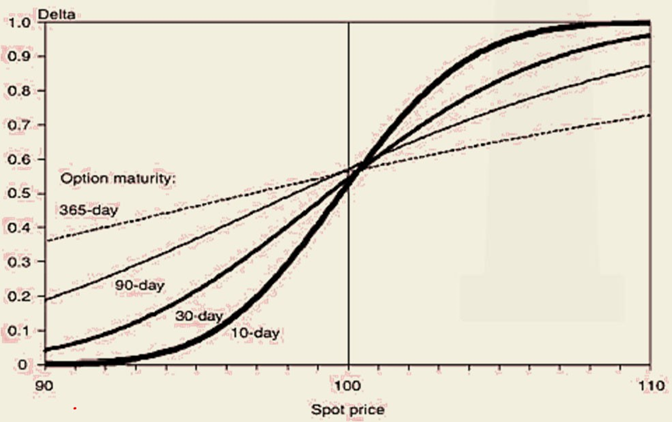
For instance, if the risk horizon is 1 day, the worst down move in the spot price is
leading to a worst price of $97.92. With a 90-day option, delta changes from 0.536 to 0.452 only. With such a small change, the linear effect will dominate the nonlinear effect. Thus linear approximations may be acceptable for options with long maturities when the risk horizon is short.
- In the extreme case, where the option is deep in the money, both 𝑁(𝑑1) and 𝑁(𝑑2) are equal to unity, and the option behaves exactly like a position in a forward contract. In this case, the BS model reduces to 𝑐 = 𝑆𝑒–r∗c — 𝐾𝑒–rc, which is indeed the valuation formula for a forward contract. Also note that the position on the dollar bill 𝐾𝑒–rc𝑁 𝑑2 is equivalent to
This shows that the call option is equivalent to a position of Δ in the underlying asset plus a short position of Δ𝑆 — 𝑐 in a dollar bill, that is
𝐿𝑜𝑛𝑔 𝑜𝑝𝑡𝑖𝑜𝑛 = 𝑙𝑜𝑛𝑔 Δ 𝑎𝑠𝑠𝑒𝑡 + 𝑠ℎ𝑜𝑟𝑡 Δ𝑆 — 𝑐 𝑏𝑖𝑙𝑙
For example, assume that the delta for an at-the-money call option on an asset worth $100 is Δ =
0.536. The option itself is worth $4.20. This option is equivalent to a ΔS = $53.60 position in the underlying asset financed by a loan of ΔS – c = $53.60 – $4.20 = $49.40.
- The next step in the risk measurement process is the aggregation of exposures across the portfolio. Thus all options on the same underlying risk factor are decomposed into their delta equivalents, which are summed across the portfolio. This generalizes to movements in the implied volatility, if necessary. The option portfolio would be characterized by its net vega, or Λ. This decomposition also can take into account second-order derivatives using the net gamma, or Γ. These exposures can be combined with simulations of the underlying risk factors to generate a risk distribution.
- Exact allocation of exposures on the risk factors. For Example : This is obtained for derivatives, when the price is an exact function of the risk factors. The partial derivatives of the price function generate analytical measures of exposures on the risk factors.
- Estimated exposures. For Example: This occurs, when a stock is replaced by a position in the stock index. The exposure then is estimated by the slope coefficient from a regression of the stock return on the index return.
General and Specific Risks
- The choice of the number of primitive (or general market) risk factors reflects the tradeoff between better quality of the approximation and faster processing.
- More factors will require more time devoted to the modelling process and risk computation.2. More factors will lead to tighter risk measurement, and hence, it may result in better
- approximation of the risk exposure of the position.
- The choice of primitive risk factors also influences the size of specific risks. Specific risk can be defined as risk that is due to issuer-specific price movements, after accounting for general market factors.
- The Basel rules have a separate charge for specific risk. Typically, the charge is 4 percent of the position value for equities and unrated debt, assuming that the banks’ models do not incorporate specific risks.
- To illustrate this decomposition, consider a portfolio of 𝑁 stocks. We are mapping each stock on a position in the stock market index, which is our primitive risk factor. The return on a stock 𝑅i is regressed on the return on the stock market index 𝑅m, i.e.,
α, which does not contribute to risk, is ignored. We assume that the specific risk owing to 𝜖 is not correlated across stocks or with the market. The relative weight of each stock in the portfolio is given by 𝑤i. Thus the portfolio return is
where 𝛽P = Beta of the portfolio, which is the weighted average of the individual betas
Now, let’s decompose the variance of the portfolio return
The first component is the general market risk. The second component is the aggregate of specific risk for the entire portfolio. This decomposition shows that with more detail on the primitive or general-market risk factors, there will be less specific risk for a fixed amount of total risk 𝑉𝑎𝑟(𝑅p).
So if there are two identical portfolios of stocks, where one is mapped to only one general risk factor, and the other is mapped to many general risk factors, then the specific risk for the second portfolio will be lesser.
- We have to restrict the number of risk factors to a small set. For some portfolios, one risk factor may be sufficient. For others, many may be necessary. For option portfolios, we need to model movements in their implied volatilities as well, apart from yields
- For a portfolio of bonds, the primitive risk factors could be movements in a set of 𝐽 government bond yields 𝑧j and in a set of 𝐾 credit spreads 𝑠k sorted by credit rating. We model the movement in each corporate bond yield 𝑑𝑦i, by a movement in z at the closest maturity and in 𝑠 for the same credit rating. The remaining component is si
The movement in the value, say 𝑊 then is
where 𝐷𝑉𝐵𝑃 is the total dollar value of a basis point for the associated risk factor.
This leads to a total risk decomposition of
A greater number of general risk factors should create less residual risk. In practice, there may not be sufficient history to measure the specific risk of individual bonds, which is why i is often assumed that all issuers within the same risk class have the same risk.
Three Approaches For Fixed Income Portfolios
- The three approaches to mapping portfolios of fixed income securities are:
- Principal mapping – One risk factor is chosen that corresponds to the average portfolio maturity. Coupon is stripped out and only principal repayment is considered at maturity.
- Duration mapping – One risk factor is chosen that corresponds to the portfolio duration. Coupons are incorporated along with the principal.
- Cash flow mapping – Several risk factor are chosen and the bond risk is decomposed into risk of each of the bond cash flows. The portfolio cash flows are grouped into maturity buckets. Cash flow mapping is the most precise method because present values of the cash flows are considered, and inter-maturity correlations are included.
Mapping Fixed Income Portfolios
| Term | Yield | Yield VaR | Modified Duration | Returns VaR |
|---|---|---|---|---|
| 1 | 5.830% | 0.00497401 | 0.944911651 | 0.470% |
| 2 | 5.710% | 0.005216789 | 1.891968593 | 0.987% |
| 3 | 5.810% | 0.005234068 | 2.835270768 | 1.484% |
| 4 | 5.890% | 0.00521773 | 3.777504958 | 1.971% |
| 5 | 5.960% | 0.005141179 | 4.718761797 | 2.426% |
| 7 | 6.070% | 0.004836792 | 6.59941548 | 3.192% |
| 9 | 6.200% | 0.00462442 | 8.474576271 | 3.919% |
| 10 | 6.260% | 0.00451605 | 9.410878976 | 4.250% |
| Term (Year) | Cash Flows | Spot Rate | Mapping (PV) | |||
|---|---|---|---|---|---|---|
| 5 Year Bond | 1 Year Bond | Principal | Duration | Cash Flow | ||
| 1 | $6 | $104 | 4.000% | 0 | 0 | $105.77 |
| 2 | $6 | 0 | 4.618% | 0 | 0 | $5.48 |
| 2.733 | — | — | — | — | $200 | — |
| 3 | $6 | 0 | 5.192% | $200 | 0 | $5.15 |
| 4 | $6 | 0 | 5.716% | 0 | 0 | $4.80 |
| 5 | $106 | 0 | 6.112% | 0 | 0 | $78.79 |
| Total | — | — | $200 | $200 | $200 | |
Mapping Fixed Income Portfolios-CF Mapping
| Term (Year) | PV Cash Flows | Individual VaR | Correlation Matrix R | Component VaR | |||||
|---|---|---|---|---|---|---|---|---|---|
| x | x × V | 1Y | 2Y | 3Y | 4Y | 5Y | (xΔVaR) | ||
| 1 | $105.77 | 0.4966 | 1 | – | – | – | – | $0.45 | |
| 2 | $5.48 | 0.0540 | 0.897 | 1 | – | – | – | $0.05 | |
| 3 | $5.15 | 0.0765 | 0.886 | 0.991 | 1 | – | – | $0.08 | |
| 4 | $4.80 | 0.0947 | 0.866 | 0.976 | 0.994 | 1 | – | $0.09 | |
| 5 | $78.79 | 1.9115 | 0.855 | 0.966 | 0.988 | 0.998 | 1 | $1.90 | |
| Total | $200.00 | 2.6335 | |||||||
| Undiversified VaR | $2.63 | ||||||||
| Diversified VaR | $2.57 | ||||||||
Three Approaches For Fixed Income Portfolios
- Cash Flow mapping VaR is less than the duration VaR because of two reasons :
- First, risk measures are not perfectly linear with maturity
- Second, correlations are below unity, which reduces risk even further.
- Out of the total $130,000 difference ($2.70 million – $2.57 million) in these measures, $70,000 ($2.70-$2.63 million) is due to differences in yield volatility, and the remaining $60,000 is due to imperfect correlations.
Stress Testing
COMPUTING VAR FROM CHANGE IN PRICE OF ZEROS
| Term (Year) | Cash Flows | Spot Rate | Old Discount Factor | PV Of CF | Risk (%) | New Discount Factor | New PV of CF |
|---|---|---|---|---|---|---|---|
| 1 | $110 | 4.000% | 0.96154 | $105.769 | 0.470% | 0.95702 | $105.272 |
| 2 | $6 | 4.618% | 0.91367 | $5.482 | 0.987% | 0.90465 | $5.428 |
| 3 | $6 | 5.192% | 0.85912 | $5.155 | 1.484% | 0.84637 | $5.078 |
| 4 | $6 | 5.716% | 0.80064 | $4.804 | 1.971% | 0.78486 | $4.709 |
| 5 | $106 | 6.112% | 0.74332 | $78.792 | 2.426% | 0.72529 | $76.881 |
| Total | $200.00 | $197.368 | |||||
| Loss | $2.634 | ||||||
Benchmarking
| Vertex | Risk (%) | Position: Index ($) | Position: Portfolio | ||||
|---|---|---|---|---|---|---|---|
| 1 ($) | 2 ($) | 3 ($) | 4 ($) | 5 ($) | |||
| ≤1m | 0.022 | 1.05 | 0.0 | 0.0 | 0.0 | 0.0 | 84.8 |
| 3m | 0.065 | 1.35 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 6m | 0.163 | 2.49 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 1Y | 0.470 | 13.96 | 0.0 | 0.0 | 59.8 | 0.0 | 0.0 |
| 2Y | 0.987 | 24.83 | 0.0 | 62.6 | 0.0 | 0.0 | 0.0 |
| 3Y | 1.484 | 15.40 | 0.0 | 59.5 | 0.0 | 0.0 | 0.0 |
| 4Y | 1.971 | 11.57 | 38.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 5Y | 2.426 | 7.62 | 62.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 7Y | 3.192 | 6.43 | 0.0 | 40.5 | 0.0 | 0.0 | 0.0 |
| 9Y | 3.913 | 4.51 | 0.0 | 37.4 | 0.0 | 0.0 | 0.0 |
| 10Y | 4.250 | 3.34 | 0.0 | 0.0 | 40.2 | 0.0 | 0.0 |
| 15Y | 6.234 | 3.00 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 20Y | 8.146 | 3.15 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 30Y | 11.119 | 1.31 | 0.0 | 0.0 | 0.0 | 0.0 | 15.2 |
| Total | 100.00 | 100.00 | |||||
| Duration | 4.62 | ||||||
| Absolute VaR | $1.99 $2.25 $2.16 $2.04 $1.94 $1.71 | ||||||
| Tracking error VaR | $0.00 $0.43 $0.29 $0.16 $0.20 $0.81 | ||||||
This table presents the cash-flow decomposition of the J.P. Morgan U.S. bond index, which has a duration of 4.62 years. Assume that we are trying to benchmark a portfolio of $100 million. Over a monthly horizon, the VaR of the index at the 95 percent confidence level is $1.99 million. This is about equivalent to the risk of a 4-year note.
- We try to match the index with two bonds. The rightmost columns in the table display the positions of two-bond portfolios with duration matched to that of the index. Since no zero- coupon has a maturity of exactly 4.62 years, the closest portfolio consists of two positions, each in a 4- and a 5-year zero. The respective weights for this portfolio are $38 million and $62 million.
If we define the new vector of positions for this portfolio as 𝑥 and for the index as 𝑥0. The VaR of the deviation relative to the benchmark is
Mapping Forwards
- From Part 1, we know that if we assume continuous compounding, then the current value of the forward contract is
And for discrete compounding it can be written as
For currency forwards, the foreign interest rate will take the place of 𝑦 in the above equations.
Apart from that it can also be expressed as the is the present value of the difference between the current forward rate and the locked-in delivery rate, i.e.
So, if we are long a forward contract with contracted rate 𝐾, we can liquidate the contract by entering a new contract to sell at the current rate 𝐹t. This will lock in a profit of (𝐹t – 𝐾), which we need to discount to the present time to find 𝐹t .
Risk and Correlations for Forward Contract Risk Factors (monthly VaR at 95 percent level)
| Risk Factor | Price or Rate | VaR (%) | Correlations | ||
|---|---|---|---|---|---|
| EUR Spot | EUR 1Y | USD 1Y | |||
| EUR spot | $1.2877 | 0.0400 | |||
| Long EUR bill | 2.2810% | -0.0583 | |||
| Short EUR bill | 3.3304% | 0.2121 | 0.0400 | -0.0583 | 1 |
| EUR forward | $1.3009 | ||||
- This table examines the risk of a 1-year forward contract to purchase 100 million euros in exchange for $130.086 million. The first step is to find the market value of the contract using the previous equation for discrete compounding
Thus the initial value of the contract is zero. This value may change, creating market risk.
- Among the three sources of risk, the volatility of the spot contract is the highest by far, with a 4.54 percent VaR (corresponding to 1.65 standard deviations over a month for a 95 percent confidence level). This is much greater than the 0.14 percent VaR for the EUR 1-year bill or even the 0.21 percent VaR for the USD bill. Thus most of the risk of the forward contract is driven by the cash EUR position.
- But risk is also affected by correlations. The positive correlation of 0.13 between the EUR spot and bill positions indicates that when the EUR goes up in value against the dollar, the value of a 1-year EUR investment is likely to appreciate. Therefore, higher values of the EUR are associated with lower EUR interest rates. This positive correlation increases the risk of the combined position. On the other hand, the position is also short a 1-year USD bill, which is correlated with the other two legs of the transaction. The issue is, what will be the net effect on the risk of the forward contract?
Computing VaR for a EUR 100 Million Forward Contract (monthly VaR at 95 percent level)
| Position | Present-value Factor | Cash Flow (CF) | PV of Flows, \(x\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| EUR spot | — | $125.89 | $5.713 | $5.704 | |
| Long EUR bill | 0.977698 | EUR100.00 | $125.89 | $0.176 | $0.029 |
| Short EUR bill | 0.967769 | —130.09 | —125.89 | $0.267 | $0.002 |
| Undiversified VaR | — | $6.156 | — | ||
| Diversified VaR | — | — | $5.735 | ||
- A long position in a forward contract that is used to purchase foreign currency with USD, one year from now, has three components:
- Long position in foreign currency spot i.e. a long spot position in EUR, worth EUR 100 million = $130.09 million in a year, or ((𝑆𝑒–rc) = $125.89 million now
- Long position in foreign currency bill i.e. a long position in a EUR investment, also worth $125.89 million now,
- Short position in USD bill i.e. a short position in a USD investment, worth $130.09 million i a year, or (𝐾𝑒–rc) = $125.89 million now.
- Considering only the spot position, the VaR is $125.89 million times the risk of 4.538 percent, which is $5.713 million. To compute the diversified VaR, we use the risk matrix from the data in the previous table and pre- and post-multiply by the vector of positions (PV of flows column in the table). The total VaR for the forward contract is $5.735 million. This number is about the same size as that of the spot contract because exchange-rate volatility dominates the volatility of 1-year bonds.
- More generally, the same methodology can be used for long-term currency swaps, which are equivalent to portfolios of forward contracts. For instance, a 10-year contract to pay dollars and receive euros is equivalent to a series of 10 forward contracts to exchange a set amount of dollars into marks. To compute the VaR, the contract must be broken down into a currency-risk component and a string of USD and EUR fixed-income components. As before, the total VaR will be driven primarily by the currency component.
Mapping Commodity Forwards
- The valuation of forward or futures contracts on commodities is substantially more complex than for financial assets such as currencies, bonds, or stock indices. Most commodities do not make monetary payments but instead are consumed, thus creating an implied benefit. This flow of benefit, net of storage cost, is loosely called convenience yield to represent the benefit from holding the cash product. This convenience yield, however, is not tied to another financial variable, such as the foreign interest rate for currency futures. It is also highly variable, creating its own source of risk.
- Monthly VaR measures for commodity futures are very high, in contrast to currency and equity market VaRs. Thus commodities are much more volatile than typical financial assets.
- Volatilities decrease with maturity of commodity futures contracts. The effect is strongest for less storable products such as energy products and less so for base metals. And this effect is very light for precious metals, which have low storage costs and no convenience yield. For financial assets, volatilities are driven primarily by spot prices, which implies basically constant volatilities across contract maturities.
- The forward rate agreement can be decomposed into two zero coupon components. If long position in a 6 x 12 FRA is considered, the components are:
- Long position in a 6-month bill
- Short position in a 12-month bill
Computing the VaR of a $100 FRA (monthly VaR at 95 percent level)
| Position | PV of Flows \(x\) | Risk % \(V\) | Correlation Matrix \(R\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| 180 days | —$97.264 | 0.1629 | 1 0.8738 |
$0.158 | $5.704 |
| 360 days | $97.264 | 0.4696 | 0.8738 1 |
$0.457 | $0.029 |
| Undiversified VaR | — | $0.615 | — | ||
| Diversified VaR | — | — | $0.327 | ||
his table provides a worked-out example. If the 360-day spot rate is 5.8125 percent and the 180-day rate is 5.6250 percent, the forward rate must be such that
The present value of the notional $100 million in 6 months is
This amount is invested for 12 months.
Mapping Forward Rate Agreements
- The previous table also displays the computation of VaR for the FRA. The VaRs of 6- and 12-month zeroes are 0.1629 and 0.4696, respectively, with a correlation of 0.8738. Applied to the principal of $97.26 million, the individual VaRs are $0.158 million and $0.457 million, which gives an undiversified VaR of $0.615 million. Fortunately, the correlation substantially lowers the FRA risk. The largest amount the position can lose over a month at the 95 percent level is $0.327 million.
Mapping Interest Rate Swap
- Interest-rate swaps are the most actively used derivatives. They create exchanges of interest- rate flows from fixed to floating or vice versa. Swaps can be decomposed into two legs, a fixed leg and a floating leg. The fixed leg can be priced as a coupon-paying bond; the floating leg is equivalent to a floating-rate note (FRN).
- To illustrate, let us compute the VaR of a $100 million 5-year interest-rate swap. We enter a dollar swap that pays 6.195 percent annually for 5 years in exchange for floating-rate payments indexed to London Interbank Offer Rate (LIBOR). Initially, we consider a situation where the floating-rate note is about to be reset. Just before the reset period, we know that the coupon will be set at the prevailing market rate. Therefore, the note carries no market risk, and its value can be mapped on cash only. Right after the reset, however, the note becomes similar to a bill with maturity equal to the next reset period.
- Interest-rate swaps can be viewed in two different ways: as (1) a combined position in a fixed- rate bond and in a floating-rate bond or (2) a portfolio of forward contracts. We first value the swap as a position in two bonds using risk VaR data from the earlier table that we used to map fixed income securities. The analysis is detailed in table given in the next slide.
Computing the VaR of a $100 million Interest Rate Swap (monthly VaR at 95 percent level)
| Term (Year) | Cash Flow | Spot Rate | PV of Net Cash Flow | Individual VaR | Component VaR | |
|---|---|---|---|---|---|---|
| Fixed | Floating | |||||
| 0 | $0 | +100 | —$100.000 | $0 | $0 | |
| 1 | —$6.195 | $0 | 5.813% | —$5.855 | $0.027 | $0.024 |
| 2 | —$6.195 | $0 | 5.929% | —$5.521 | $0.054 | $0.053 |
| 3 | —$6.195 | $0 | 6.034% | —$5.196 | $0.077 | $0.075 |
| 4 | —$6.195 | $0 | 6.130% | —$4.833 | $0.096 | $0.096 |
| 5 | —$106.195 | $0 | 6.217% | —$78.546 | $1.905 | $1.905 |
| Total | $0.000 | |||||
| Undiversified VaR | $2.160 | |||||
| Diversified VaR | $2.152 | |||||
- The second and third columns lay out the payments on both legs. Just before reset, the long position in the FRN is worth $100 million, and the market value of the swap is zero. To clarify the allocation of current values, the FRN is allocated to cash, with a zero maturity. This has no risk. The next column lists the zero-coupon swap rates for maturities going from 1 to 5 years. The fifth column reports the present value of the net cash flows, fixed minus floating. The last column presents the component VaR, which adds up to a total diversified VaR of $2.152 million. The undiversified VaR is obtained from summing all individual VaRs. As usual, the $2.160 millio value somewhat overestimates risk.
- This swap can also be viewed as the sum of five forward contracts, as shown in this table. The 1-year contract promises payment of $100 million plus the coupon of 6.195 percent; discounted at the spot rate of 5.813 percent, this yields a present value of -$100.36 million. This is in exchange for $100 million now, which has no risk.
An Interest-Rate Swap Viewed as Forward Contracts (monthly VaR at 95 percent level)
| Term (Year) | PV of Flows: Contract | VaR | ||||
|---|---|---|---|---|---|---|
| 1 | 1 × 2 | 2 × 3 | 3 × 4 | 4 × 5 | ||
| 1 | —$100.36 | $94.50 | ||||
| 2 | -$94.64 | $89.11 | ||||
| 3 | $89.08 | $83.88 | ||||
| 4 | -$83.70 | $78.82 | ||||
| 5 | —$78.55 | |||||
| VaR | $0.471 | $0.571 | $0.488 | $0.446 | $0.425 | |
| Undiversified VaR | $2.401 | |||||
| Diversified VaR | $2.152 | |||||
- The next contract is a 1 x 2 forward contract that promises to pay the principal plus the fixed coupon in 2 years, or -$106.195 million; discounted at the 2-year spot rate, this yields -$94.64 million. This is in exchange for $100 million in 1 year, which is also $94.50 million when discounted at the 1-year spot rate. And so on until the fifth contract, a 4 x 5 forward contract.
- The previous table also shows the VaR of each contract. The undiversified VaR of $2.401 million is the result of a simple summation of the five VaRs. The fully diversified VaR is
- $2.152 million, exactly the same as in the preceding table. This demonstrates the equivalence of the two approaches.
- Finally, we examine the change in risk after the first payment has just been set on the floating- rate leg. The FRN then becomes a 1-year bond initially valued at par but subject to fluctuations in rates. The only change in the pattern of cash flows in Table 4-10 is to add $100 million to the position on year 1 (from -$5.855 to $94.145). The resulting VaR then decreases from $2.152 million to $1.763 million. More generally, the swap’s VaR will converge to zero as the swap matures, dipping each time a coupon is set.
Mapping Options
- Let’s consider the mapping process for nonlinear derivatives, or options. Obviously, this nonlinearity may create problems for risk measurement systems based on the delta-normal approach, which is fundamentally linear. To simplify, consider the Black-Scholes (BS) model for European options. From Part 1, we remember that
where 𝑁(𝑑) is the cumulative normal distribution function with arguments, and
where 𝐾 is now the exercise price at which the option holder can, but is not obligated to, buy the asset.
- Delta, is particularly important for options. For a European call, this is
. This figure displays its behavior as a function of the underlying spot price and for various maturities. The figure shows that delta is not a constant, which may make linear methods inappropriate for measuring the risk of options. Delta increases with the underlying spot price. The relationship becomes more nonlinear for short-term options, for example, with an option maturity of 10 days. Linear methods approximate delta by a constant value over the risk horizon. The quality of this approximation depends on parameter values.

For instance, if the risk horizon is 1 day, the worst down move in the spot price is
leading to a worst price of $97.92. With a 90-day option, delta changes from 0.536 to 0.452 only. With such a small change, the linear effect will dominate the nonlinear effect. Thus linear approximations may be acceptable for options with long maturities when the risk horizon is short.
- In the extreme case, where the option is deep in the money, both 𝑁(𝑑1) and 𝑁(𝑑2) are equal to unity, and the option behaves exactly like a position in a forward contract. In this case, the BS model reduces to 𝑐 = 𝑆𝑒–r∗c — 𝐾𝑒–rc, which is indeed the valuation formula for a forward contract. Also note that the position on the dollar bill 𝐾𝑒–rc𝑁 𝑑2 is equivalent to
This shows that the call option is equivalent to a position of Δ in the underlying asset plus a short position of Δ𝑆 — 𝑐 in a dollar bill, that is
𝐿𝑜𝑛𝑔 𝑜𝑝𝑡𝑖𝑜𝑛 = 𝑙𝑜𝑛𝑔 Δ 𝑎𝑠𝑠𝑒𝑡 + 𝑠ℎ𝑜𝑟𝑡 Δ𝑆 — 𝑐 𝑏𝑖𝑙𝑙
For example, assume that the delta for an at-the-money call option on an asset worth $100 is Δ =
0.536. The option itself is worth $4.20. This option is equivalent to a ΔS = $53.60 position in the underlying asset financed by a loan of ΔS – c = $53.60 – $4.20 = $49.40.
- The next step in the risk measurement process is the aggregation of exposures across the portfolio. Thus all options on the same underlying risk factor are decomposed into their delta equivalents, which are summed across the portfolio. This generalizes to movements in the implied volatility, if necessary. The option portfolio would be characterized by its net vega, or Λ. This decomposition also can take into account second-order derivatives using the net gamma, or Γ. These exposures can be combined with simulations of the underlying risk factors to generate a risk distribution.
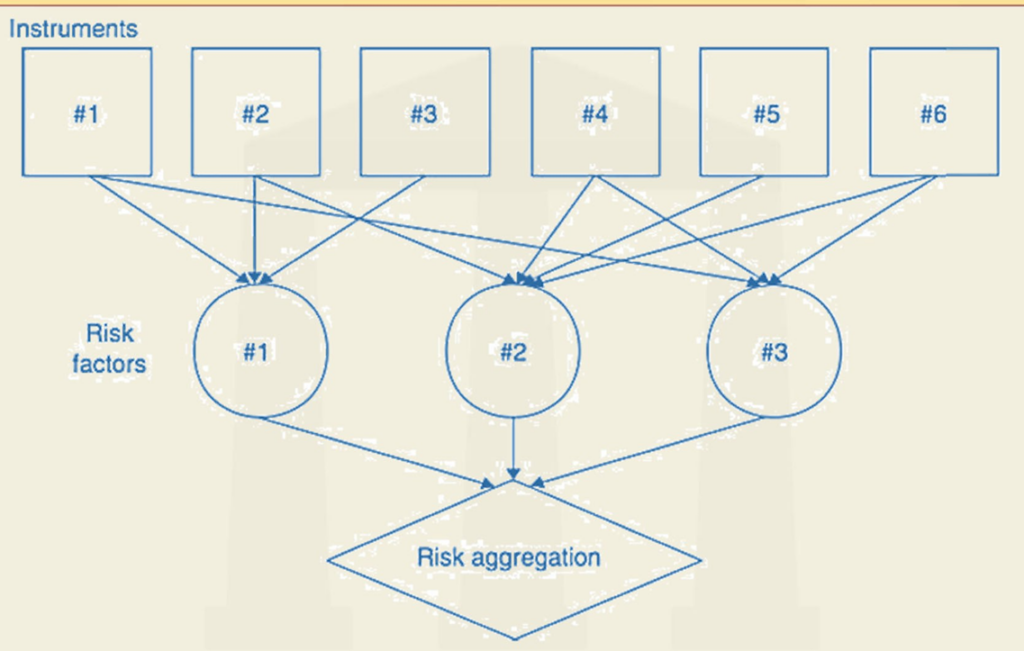
| Market Value | Exposure on Risk Factor | |||
|---|---|---|---|---|
| 1 | 2 | 3 | ||
| Instrument 1 | V1 | x11 | x12 | x13 |
| Instrument 2 | V2 | x21 | x22 | x23 |
| Instrument 3 | V3 | x31 | x32 | x33 |
| Instrument 4 | V4 | x41 | x42 | x43 |
| Instrument 5 | V5 | x51 | x52 | x53 |
| Instrument 6 | V6 | x61 | x62 | x63 |
| Total Portfolio | V | |||
- Two kinds of mapping include:
-
- Exact allocation of exposures on the risk factors. For Example : This is obtained for derivatives, when the price is an exact function of the risk factors. The partial derivatives of the price function generate analytical measures of exposures on the risk factors.
- Estimated exposures. For Example: This occurs, when a stock is replaced by a position in the stock index. The exposure then is estimated by the slope coefficient from a regression of the stock return on the index return.
General and Specific Risks
- The choice of the number of primitive (or general market) risk factors reflects the tradeoff between better quality of the approximation and faster processing.
- More factors will require more time devoted to the modelling process and risk computation.2. More factors will lead to tighter risk measurement, and hence, it may result in better
- approximation of the risk exposure of the position.
- The choice of primitive risk factors also influences the size of specific risks. Specific risk can be defined as risk that is due to issuer-specific price movements, after accounting for general market factors.
- The Basel rules have a separate charge for specific risk. Typically, the charge is 4 percent of the position value for equities and unrated debt, assuming that the banks’ models do not incorporate specific risks.
- To illustrate this decomposition, consider a portfolio of 𝑁 stocks. We are mapping each stock on a position in the stock market index, which is our primitive risk factor. The return on a stock 𝑅i is regressed on the return on the stock market index 𝑅m, i.e.,
α, which does not contribute to risk, is ignored. We assume that the specific risk owing to 𝜖 is not correlated across stocks or with the market. The relative weight of each stock in the portfolio is given by 𝑤i. Thus the portfolio return is
where 𝛽P = Beta of the portfolio, which is the weighted average of the individual betas
Now, let’s decompose the variance of the portfolio return
The first component is the general market risk. The second component is the aggregate of specific risk for the entire portfolio. This decomposition shows that with more detail on the primitive or general-market risk factors, there will be less specific risk for a fixed amount of total risk 𝑉𝑎𝑟(𝑅p).
So if there are two identical portfolios of stocks, where one is mapped to only one general risk factor, and the other is mapped to many general risk factors, then the specific risk for the second portfolio will be lesser.
- We have to restrict the number of risk factors to a small set. For some portfolios, one risk factor may be sufficient. For others, many may be necessary. For option portfolios, we need to model movements in their implied volatilities as well, apart from yields
- For a portfolio of bonds, the primitive risk factors could be movements in a set of 𝐽 government bond yields 𝑧j and in a set of 𝐾 credit spreads 𝑠k sorted by credit rating. We model the movement in each corporate bond yield 𝑑𝑦i, by a movement in z at the closest maturity and in 𝑠 for the same credit rating. The remaining component is si
The movement in the value, say 𝑊 then is
where 𝐷𝑉𝐵𝑃 is the total dollar value of a basis point for the associated risk factor.
This leads to a total risk decomposition of
A greater number of general risk factors should create less residual risk. In practice, there may not be sufficient history to measure the specific risk of individual bonds, which is why i is often assumed that all issuers within the same risk class have the same risk.
Three Approaches For Fixed Income Portfolios
- The three approaches to mapping portfolios of fixed income securities are:
- Principal mapping – One risk factor is chosen that corresponds to the average portfolio maturity. Coupon is stripped out and only principal repayment is considered at maturity.
- Duration mapping – One risk factor is chosen that corresponds to the portfolio duration. Coupons are incorporated along with the principal.
- Cash flow mapping – Several risk factor are chosen and the bond risk is decomposed into risk of each of the bond cash flows. The portfolio cash flows are grouped into maturity buckets. Cash flow mapping is the most precise method because present values of the cash flows are considered, and inter-maturity correlations are included.
Mapping Fixed Income Portfolios
| Term | Yield | Yield VaR | Modified Duration | Returns VaR |
|---|---|---|---|---|
| 1 | 5.830% | 0.00497401 | 0.944911651 | 0.470% |
| 2 | 5.710% | 0.005216789 | 1.891968593 | 0.987% |
| 3 | 5.810% | 0.005234068 | 2.835270768 | 1.484% |
| 4 | 5.890% | 0.00521773 | 3.777504958 | 1.971% |
| 5 | 5.960% | 0.005141179 | 4.718761797 | 2.426% |
| 7 | 6.070% | 0.004836792 | 6.59941548 | 3.192% |
| 9 | 6.200% | 0.00462442 | 8.474576271 | 3.919% |
| 10 | 6.260% | 0.00451605 | 9.410878976 | 4.250% |
| Term (Year) | Cash Flows | Spot Rate | Mapping (PV) | |||
|---|---|---|---|---|---|---|
| 5 Year Bond | 1 Year Bond | Principal | Duration | Cash Flow | ||
| 1 | $6 | $104 | 4.000% | 0 | 0 | $105.77 |
| 2 | $6 | 0 | 4.618% | 0 | 0 | $5.48 |
| 2.733 | — | — | — | — | $200 | — |
| 3 | $6 | 0 | 5.192% | $200 | 0 | $5.15 |
| 4 | $6 | 0 | 5.716% | 0 | 0 | $4.80 |
| 5 | $106 | 0 | 6.112% | 0 | 0 | $78.79 |
| Total | — | — | $200 | $200 | $200 | |
Mapping Fixed Income Portfolios-CF Mapping
| Term (Year) | PV Cash Flows | Individual VaR | Correlation Matrix R | Component VaR | |||||
|---|---|---|---|---|---|---|---|---|---|
| x | x × V | 1Y | 2Y | 3Y | 4Y | 5Y | (xΔVaR) | ||
| 1 | $105.77 | 0.4966 | 1 | – | – | – | – | $0.45 | |
| 2 | $5.48 | 0.0540 | 0.897 | 1 | – | – | – | $0.05 | |
| 3 | $5.15 | 0.0765 | 0.886 | 0.991 | 1 | – | – | $0.08 | |
| 4 | $4.80 | 0.0947 | 0.866 | 0.976 | 0.994 | 1 | – | $0.09 | |
| 5 | $78.79 | 1.9115 | 0.855 | 0.966 | 0.988 | 0.998 | 1 | $1.90 | |
| Total | $200.00 | 2.6335 | |||||||
| Undiversified VaR | $2.63 | ||||||||
| Diversified VaR | $2.57 | ||||||||
Three Approaches For Fixed Income Portfolios
- Cash Flow mapping VaR is less than the duration VaR because of two reasons :
- First, risk measures are not perfectly linear with maturity
- Second, correlations are below unity, which reduces risk even further.
- Out of the total $130,000 difference ($2.70 million – $2.57 million) in these measures, $70,000 ($2.70-$2.63 million) is due to differences in yield volatility, and the remaining $60,000 is due to imperfect correlations.
Stress Testing
COMPUTING VAR FROM CHANGE IN PRICE OF ZEROS
| Term (Year) | Cash Flows | Spot Rate | Old Discount Factor | PV Of CF | Risk (%) | New Discount Factor | New PV of CF |
|---|---|---|---|---|---|---|---|
| 1 | $110 | 4.000% | 0.96154 | $105.769 | 0.470% | 0.95702 | $105.272 |
| 2 | $6 | 4.618% | 0.91367 | $5.482 | 0.987% | 0.90465 | $5.428 |
| 3 | $6 | 5.192% | 0.85912 | $5.155 | 1.484% | 0.84637 | $5.078 |
| 4 | $6 | 5.716% | 0.80064 | $4.804 | 1.971% | 0.78486 | $4.709 |
| 5 | $106 | 6.112% | 0.74332 | $78.792 | 2.426% | 0.72529 | $76.881 |
| Total | $200.00 | $197.368 | |||||
| Loss | $2.634 | ||||||
Benchmarking
| Vertex | Risk (%) | Position: Index ($) | Position: Portfolio | ||||
|---|---|---|---|---|---|---|---|
| 1 ($) | 2 ($) | 3 ($) | 4 ($) | 5 ($) | |||
| ≤1m | 0.022 | 1.05 | 0.0 | 0.0 | 0.0 | 0.0 | 84.8 |
| 3m | 0.065 | 1.35 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 6m | 0.163 | 2.49 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 1Y | 0.470 | 13.96 | 0.0 | 0.0 | 59.8 | 0.0 | 0.0 |
| 2Y | 0.987 | 24.83 | 0.0 | 62.6 | 0.0 | 0.0 | 0.0 |
| 3Y | 1.484 | 15.40 | 0.0 | 59.5 | 0.0 | 0.0 | 0.0 |
| 4Y | 1.971 | 11.57 | 38.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 5Y | 2.426 | 7.62 | 62.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 7Y | 3.192 | 6.43 | 0.0 | 40.5 | 0.0 | 0.0 | 0.0 |
| 9Y | 3.913 | 4.51 | 0.0 | 37.4 | 0.0 | 0.0 | 0.0 |
| 10Y | 4.250 | 3.34 | 0.0 | 0.0 | 40.2 | 0.0 | 0.0 |
| 15Y | 6.234 | 3.00 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 20Y | 8.146 | 3.15 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 30Y | 11.119 | 1.31 | 0.0 | 0.0 | 0.0 | 0.0 | 15.2 |
| Total | 100.00 | 100.00 | |||||
| Duration | 4.62 | ||||||
| Absolute VaR | $1.99 $2.25 $2.16 $2.04 $1.94 $1.71 | ||||||
| Tracking error VaR | $0.00 $0.43 $0.29 $0.16 $0.20 $0.81 | ||||||
This table presents the cash-flow decomposition of the J.P. Morgan U.S. bond index, which has a duration of 4.62 years. Assume that we are trying to benchmark a portfolio of $100 million. Over a monthly horizon, the VaR of the index at the 95 percent confidence level is $1.99 million. This is about equivalent to the risk of a 4-year note.
- We try to match the index with two bonds. The rightmost columns in the table display the positions of two-bond portfolios with duration matched to that of the index. Since no zero- coupon has a maturity of exactly 4.62 years, the closest portfolio consists of two positions, each in a 4- and a 5-year zero. The respective weights for this portfolio are $38 million and $62 million.
If we define the new vector of positions for this portfolio as 𝑥 and for the index as 𝑥0. The VaR of the deviation relative to the benchmark is
Mapping Forwards
- From Part 1, we know that if we assume continuous compounding, then the current value of the forward contract is
And for discrete compounding it can be written as
For currency forwards, the foreign interest rate will take the place of 𝑦 in the above equations.
Apart from that it can also be expressed as the is the present value of the difference between the current forward rate and the locked-in delivery rate, i.e.
So, if we are long a forward contract with contracted rate 𝐾, we can liquidate the contract by entering a new contract to sell at the current rate 𝐹t. This will lock in a profit of (𝐹t – 𝐾), which we need to discount to the present time to find 𝐹t .
Risk and Correlations for Forward Contract Risk Factors (monthly VaR at 95 percent level)
| Risk Factor | Price or Rate | VaR (%) | Correlations | ||
|---|---|---|---|---|---|
| EUR Spot | EUR 1Y | USD 1Y | |||
| EUR spot | $1.2877 | 0.0400 | |||
| Long EUR bill | 2.2810% | -0.0583 | |||
| Short EUR bill | 3.3304% | 0.2121 | 0.0400 | -0.0583 | 1 |
| EUR forward | $1.3009 | ||||
- This table examines the risk of a 1-year forward contract to purchase 100 million euros in exchange for $130.086 million. The first step is to find the market value of the contract using the previous equation for discrete compounding
Thus the initial value of the contract is zero. This value may change, creating market risk.
- Among the three sources of risk, the volatility of the spot contract is the highest by far, with a 4.54 percent VaR (corresponding to 1.65 standard deviations over a month for a 95 percent confidence level). This is much greater than the 0.14 percent VaR for the EUR 1-year bill or even the 0.21 percent VaR for the USD bill. Thus most of the risk of the forward contract is driven by the cash EUR position.
- But risk is also affected by correlations. The positive correlation of 0.13 between the EUR spot and bill positions indicates that when the EUR goes up in value against the dollar, the value of a 1-year EUR investment is likely to appreciate. Therefore, higher values of the EUR are associated with lower EUR interest rates. This positive correlation increases the risk of the combined position. On the other hand, the position is also short a 1-year USD bill, which is correlated with the other two legs of the transaction. The issue is, what will be the net effect on the risk of the forward contract?
Computing VaR for a EUR 100 Million Forward Contract (monthly VaR at 95 percent level)
| Position | Present-value Factor | Cash Flow (CF) | PV of Flows, \(x\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| EUR spot | — | $125.89 | $5.713 | $5.704 | |
| Long EUR bill | 0.977698 | EUR100.00 | $125.89 | $0.176 | $0.029 |
| Short EUR bill | 0.967769 | —130.09 | —125.89 | $0.267 | $0.002 |
| Undiversified VaR | — | $6.156 | — | ||
| Diversified VaR | — | — | $5.735 | ||
- A long position in a forward contract that is used to purchase foreign currency with USD, one year from now, has three components:
- Long position in foreign currency spot i.e. a long spot position in EUR, worth EUR 100 million = $130.09 million in a year, or ((𝑆𝑒–rc) = $125.89 million now
- Long position in foreign currency bill i.e. a long position in a EUR investment, also worth $125.89 million now,
- Short position in USD bill i.e. a short position in a USD investment, worth $130.09 million i a year, or (𝐾𝑒–rc) = $125.89 million now.
- Considering only the spot position, the VaR is $125.89 million times the risk of 4.538 percent, which is $5.713 million. To compute the diversified VaR, we use the risk matrix from the data in the previous table and pre- and post-multiply by the vector of positions (PV of flows column in the table). The total VaR for the forward contract is $5.735 million. This number is about the same size as that of the spot contract because exchange-rate volatility dominates the volatility of 1-year bonds.
- More generally, the same methodology can be used for long-term currency swaps, which are equivalent to portfolios of forward contracts. For instance, a 10-year contract to pay dollars and receive euros is equivalent to a series of 10 forward contracts to exchange a set amount of dollars into marks. To compute the VaR, the contract must be broken down into a currency-risk component and a string of USD and EUR fixed-income components. As before, the total VaR will be driven primarily by the currency component.
Mapping Commodity Forwards
- The valuation of forward or futures contracts on commodities is substantially more complex than for financial assets such as currencies, bonds, or stock indices. Most commodities do not make monetary payments but instead are consumed, thus creating an implied benefit. This flow of benefit, net of storage cost, is loosely called convenience yield to represent the benefit from holding the cash product. This convenience yield, however, is not tied to another financial variable, such as the foreign interest rate for currency futures. It is also highly variable, creating its own source of risk.
- Monthly VaR measures for commodity futures are very high, in contrast to currency and equity market VaRs. Thus commodities are much more volatile than typical financial assets.
- Volatilities decrease with maturity of commodity futures contracts. The effect is strongest for less storable products such as energy products and less so for base metals. And this effect is very light for precious metals, which have low storage costs and no convenience yield. For financial assets, volatilities are driven primarily by spot prices, which implies basically constant volatilities across contract maturities.
- The forward rate agreement can be decomposed into two zero coupon components. If long position in a 6 x 12 FRA is considered, the components are:
- Long position in a 6-month bill
- Short position in a 12-month bill
Computing the VaR of a $100 FRA (monthly VaR at 95 percent level)
| Position | PV of Flows \(x\) | Risk % \(V\) | Correlation Matrix \(R\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| 180 days | —$97.264 | 0.1629 | 1 0.8738 |
$0.158 | $5.704 |
| 360 days | $97.264 | 0.4696 | 0.8738 1 |
$0.457 | $0.029 |
| Undiversified VaR | — | $0.615 | — | ||
| Diversified VaR | — | — | $0.327 | ||
his table provides a worked-out example. If the 360-day spot rate is 5.8125 percent and the 180-day rate is 5.6250 percent, the forward rate must be such that
The present value of the notional $100 million in 6 months is
This amount is invested for 12 months.
Mapping Forward Rate Agreements
- The previous table also displays the computation of VaR for the FRA. The VaRs of 6- and 12-month zeroes are 0.1629 and 0.4696, respectively, with a correlation of 0.8738. Applied to the principal of $97.26 million, the individual VaRs are $0.158 million and $0.457 million, which gives an undiversified VaR of $0.615 million. Fortunately, the correlation substantially lowers the FRA risk. The largest amount the position can lose over a month at the 95 percent level is $0.327 million.
Mapping Interest Rate Swap
- Interest-rate swaps are the most actively used derivatives. They create exchanges of interest- rate flows from fixed to floating or vice versa. Swaps can be decomposed into two legs, a fixed leg and a floating leg. The fixed leg can be priced as a coupon-paying bond; the floating leg is equivalent to a floating-rate note (FRN).
- To illustrate, let us compute the VaR of a $100 million 5-year interest-rate swap. We enter a dollar swap that pays 6.195 percent annually for 5 years in exchange for floating-rate payments indexed to London Interbank Offer Rate (LIBOR). Initially, we consider a situation where the floating-rate note is about to be reset. Just before the reset period, we know that the coupon will be set at the prevailing market rate. Therefore, the note carries no market risk, and its value can be mapped on cash only. Right after the reset, however, the note becomes similar to a bill with maturity equal to the next reset period.
- Interest-rate swaps can be viewed in two different ways: as (1) a combined position in a fixed- rate bond and in a floating-rate bond or (2) a portfolio of forward contracts. We first value the swap as a position in two bonds using risk VaR data from the earlier table that we used to map fixed income securities. The analysis is detailed in table given in the next slide.
Computing the VaR of a $100 million Interest Rate Swap (monthly VaR at 95 percent level)
| Term (Year) | Cash Flow | Spot Rate | PV of Net Cash Flow | Individual VaR | Component VaR | |
|---|---|---|---|---|---|---|
| Fixed | Floating | |||||
| 0 | $0 | +100 | —$100.000 | $0 | $0 | |
| 1 | —$6.195 | $0 | 5.813% | —$5.855 | $0.027 | $0.024 |
| 2 | —$6.195 | $0 | 5.929% | —$5.521 | $0.054 | $0.053 |
| 3 | —$6.195 | $0 | 6.034% | —$5.196 | $0.077 | $0.075 |
| 4 | —$6.195 | $0 | 6.130% | —$4.833 | $0.096 | $0.096 |
| 5 | —$106.195 | $0 | 6.217% | —$78.546 | $1.905 | $1.905 |
| Total | $0.000 | |||||
| Undiversified VaR | $2.160 | |||||
| Diversified VaR | $2.152 | |||||
- The second and third columns lay out the payments on both legs. Just before reset, the long position in the FRN is worth $100 million, and the market value of the swap is zero. To clarify the allocation of current values, the FRN is allocated to cash, with a zero maturity. This has no risk. The next column lists the zero-coupon swap rates for maturities going from 1 to 5 years. The fifth column reports the present value of the net cash flows, fixed minus floating. The last column presents the component VaR, which adds up to a total diversified VaR of $2.152 million. The undiversified VaR is obtained from summing all individual VaRs. As usual, the $2.160 millio value somewhat overestimates risk.
- This swap can also be viewed as the sum of five forward contracts, as shown in this table. The 1-year contract promises payment of $100 million plus the coupon of 6.195 percent; discounted at the spot rate of 5.813 percent, this yields a present value of -$100.36 million. This is in exchange for $100 million now, which has no risk.
An Interest-Rate Swap Viewed as Forward Contracts (monthly VaR at 95 percent level)
| Term (Year) | PV of Flows: Contract | VaR | ||||
|---|---|---|---|---|---|---|
| 1 | 1 × 2 | 2 × 3 | 3 × 4 | 4 × 5 | ||
| 1 | —$100.36 | $94.50 | ||||
| 2 | -$94.64 | $89.11 | ||||
| 3 | $89.08 | $83.88 | ||||
| 4 | -$83.70 | $78.82 | ||||
| 5 | —$78.55 | |||||
| VaR | $0.471 | $0.571 | $0.488 | $0.446 | $0.425 | |
| Undiversified VaR | $2.401 | |||||
| Diversified VaR | $2.152 | |||||
- The next contract is a 1 x 2 forward contract that promises to pay the principal plus the fixed coupon in 2 years, or -$106.195 million; discounted at the 2-year spot rate, this yields -$94.64 million. This is in exchange for $100 million in 1 year, which is also $94.50 million when discounted at the 1-year spot rate. And so on until the fifth contract, a 4 x 5 forward contract.
- The previous table also shows the VaR of each contract. The undiversified VaR of $2.401 million is the result of a simple summation of the five VaRs. The fully diversified VaR is
- $2.152 million, exactly the same as in the preceding table. This demonstrates the equivalence of the two approaches.
- Finally, we examine the change in risk after the first payment has just been set on the floating- rate leg. The FRN then becomes a 1-year bond initially valued at par but subject to fluctuations in rates. The only change in the pattern of cash flows in Table 4-10 is to add $100 million to the position on year 1 (from -$5.855 to $94.145). The resulting VaR then decreases from $2.152 million to $1.763 million. More generally, the swap’s VaR will converge to zero as the swap matures, dipping each time a coupon is set.
Mapping Options
- Let’s consider the mapping process for nonlinear derivatives, or options. Obviously, this nonlinearity may create problems for risk measurement systems based on the delta-normal approach, which is fundamentally linear. To simplify, consider the Black-Scholes (BS) model for European options. From Part 1, we remember that
where 𝑁(𝑑) is the cumulative normal distribution function with arguments, and
where 𝐾 is now the exercise price at which the option holder can, but is not obligated to, buy the asset.
- Delta, is particularly important for options. For a European call, this is
. This figure displays its behavior as a function of the underlying spot price and for various maturities. The figure shows that delta is not a constant, which may make linear methods inappropriate for measuring the risk of options. Delta increases with the underlying spot price. The relationship becomes more nonlinear for short-term options, for example, with an option maturity of 10 days. Linear methods approximate delta by a constant value over the risk horizon. The quality of this approximation depends on parameter values.

For instance, if the risk horizon is 1 day, the worst down move in the spot price is
leading to a worst price of $97.92. With a 90-day option, delta changes from 0.536 to 0.452 only. With such a small change, the linear effect will dominate the nonlinear effect. Thus linear approximations may be acceptable for options with long maturities when the risk horizon is short.
- In the extreme case, where the option is deep in the money, both 𝑁(𝑑1) and 𝑁(𝑑2) are equal to unity, and the option behaves exactly like a position in a forward contract. In this case, the BS model reduces to 𝑐 = 𝑆𝑒–r∗c — 𝐾𝑒–rc, which is indeed the valuation formula for a forward contract. Also note that the position on the dollar bill 𝐾𝑒–rc𝑁 𝑑2 is equivalent to
This shows that the call option is equivalent to a position of Δ in the underlying asset plus a short position of Δ𝑆 — 𝑐 in a dollar bill, that is
𝐿𝑜𝑛𝑔 𝑜𝑝𝑡𝑖𝑜𝑛 = 𝑙𝑜𝑛𝑔 Δ 𝑎𝑠𝑠𝑒𝑡 + 𝑠ℎ𝑜𝑟𝑡 Δ𝑆 — 𝑐 𝑏𝑖𝑙𝑙
For example, assume that the delta for an at-the-money call option on an asset worth $100 is Δ =
0.536. The option itself is worth $4.20. This option is equivalent to a ΔS = $53.60 position in the underlying asset financed by a loan of ΔS – c = $53.60 – $4.20 = $49.40.
- The next step in the risk measurement process is the aggregation of exposures across the portfolio. Thus all options on the same underlying risk factor are decomposed into their delta equivalents, which are summed across the portfolio. This generalizes to movements in the implied volatility, if necessary. The option portfolio would be characterized by its net vega, or Λ. This decomposition also can take into account second-order derivatives using the net gamma, or Γ. These exposures can be combined with simulations of the underlying risk factors to generate a risk distribution.
Principle Underlying VaR Mapping
- Mapping is the process by which the current values of the portfolio positions are replaced by exposures on the risk factors. A small number of risk factors is identified and the complex position is decomposed in terms of these factors.
NEED FOR MAPPING
“J.P. Morgan Chase’s VaR calculation is highly granular, comprising more than 2.1 million positions and 240,000 pricing series (e.g., securities prices, interest rates, foreign exchange rates).”
(Annual report, 2004)
Considering these positions separately and modelling them individually as risk factors is too complex & time-consuming.
Advantages of VaR Mapping
- A large portfolio having too many positions can be decomposed – For example, consider a trader’s desk with thousands of open dollar/ euro forward contracts. The positions may differ owing to different maturities and delivery prices. It is unnecessary, however, to model all these positions individually. Basically, the positions are exposed to a single major risk factor, which is the dollar/euro spot exchange rate. Thus they could be summarized by a single aggregate exposure on this risk factor.
- Common risk factors can be identified and risk exposures are simplified into primitive risk factors. Examples of primitive risk factors are : Zero-coupon yield, spot exchange rates, forward price etc.
- Too many computations would be avoided, and the time needed to measure risk would be reduced.
- Stress testing risk factors becomes easier.
- It provides a solution when historical data or complete data is not available. Often a complete history of all securities may not exist or may not be relevant. Consider a mutual fund with a strategy of investing in initial public offerings (IPOs) of common stock. By definition, these stocks have no history. They certainly cannot be ignored in the risk system, however. The risk manager can replace these positions by exposures on similar risk factors already in the system.
- Mapping is also helpful when the characteristics of the instrument change over time. For example the risk profile of bonds changes as they age. One cannot use the history of prices on a bond directly. Instead, the bond must be mapped on yields that best represent its current profile. Similarly, the risk profile of options changes very quickly. Options must be mapped on their primary risk factors. Mapping provides a way to tackle these practical problems
- Mapping can also be a solution to the problem of stale prices. Consider, for instance, a portfolio or mutual funds invested in international stocks. As much as 15 hours can elapse from the time the market closes in Tokyo at 1:00 A.M. EST (3:00 P.M. in Japan) to the time it closes in the United States at 4:00 P.M. As a result, prices from the Tokyo close ignore intervening information and are said to be stale. This led to the mutual-fund scandal of 2003.
Disadvantages of VaR Mapping
- Although aggregation through VaR mapping is perfectly acceptable for risk measurement purposes, but it is not appropriate for the pricing of the portfolio. So it leads to a less accurate pricing of the portfolio. This is why risk management methods can differ from pricing methods.
General Mapping Process
- The generic VaR mapping process is as follows:
- A set of elementary risk factors is identified and their probability distributions is estimated.
- Financial instruments are decomposed into exposures on these risk factors.
- Each position is marked to market.
- Market value of each instrument is allocated to risk factors. If the current market value is not fully allocated to the risk factors, it must mean that the remainder is allocated to cash, which is not a risk factor because it has no risk.
- The exposure for all positions is aggregated to build the return distribution of a portfolio, and subsequently portfolio VaR is calculated.

| Market Value | Exposure on Risk Factor | |||
|---|---|---|---|---|
| 1 | 2 | 3 | ||
| Instrument 1 | V1 | x11 | x12 | x13 |
| Instrument 2 | V2 | x21 | x22 | x23 |
| Instrument 3 | V3 | x31 | x32 | x33 |
| Instrument 4 | V4 | x41 | x42 | x43 |
| Instrument 5 | V5 | x51 | x52 | x53 |
| Instrument 6 | V6 | x61 | x62 | x63 |
| Total Portfolio | V | |||
- Two kinds of mapping include:
-
- Exact allocation of exposures on the risk factors. For Example : This is obtained for derivatives, when the price is an exact function of the risk factors. The partial derivatives of the price function generate analytical measures of exposures on the risk factors.
- Estimated exposures. For Example: This occurs, when a stock is replaced by a position in the stock index. The exposure then is estimated by the slope coefficient from a regression of the stock return on the index return.
General and Specific Risks
- The choice of the number of primitive (or general market) risk factors reflects the tradeoff between better quality of the approximation and faster processing.
- More factors will require more time devoted to the modelling process and risk computation.2. More factors will lead to tighter risk measurement, and hence, it may result in better
- approximation of the risk exposure of the position.
- The choice of primitive risk factors also influences the size of specific risks. Specific risk can be defined as risk that is due to issuer-specific price movements, after accounting for general market factors.
- The Basel rules have a separate charge for specific risk. Typically, the charge is 4 percent of the position value for equities and unrated debt, assuming that the banks’ models do not incorporate specific risks.
- To illustrate this decomposition, consider a portfolio of 𝑁 stocks. We are mapping each stock on a position in the stock market index, which is our primitive risk factor. The return on a stock 𝑅i is regressed on the return on the stock market index 𝑅m, i.e.,
α, which does not contribute to risk, is ignored. We assume that the specific risk owing to 𝜖 is not correlated across stocks or with the market. The relative weight of each stock in the portfolio is given by 𝑤i. Thus the portfolio return is
where 𝛽P = Beta of the portfolio, which is the weighted average of the individual betas
Now, let’s decompose the variance of the portfolio return
The first component is the general market risk. The second component is the aggregate of specific risk for the entire portfolio. This decomposition shows that with more detail on the primitive or general-market risk factors, there will be less specific risk for a fixed amount of total risk 𝑉𝑎𝑟(𝑅p).
So if there are two identical portfolios of stocks, where one is mapped to only one general risk factor, and the other is mapped to many general risk factors, then the specific risk for the second portfolio will be lesser.
- We have to restrict the number of risk factors to a small set. For some portfolios, one risk factor may be sufficient. For others, many may be necessary. For option portfolios, we need to model movements in their implied volatilities as well, apart from yields
- For a portfolio of bonds, the primitive risk factors could be movements in a set of 𝐽 government bond yields 𝑧j and in a set of 𝐾 credit spreads 𝑠k sorted by credit rating. We model the movement in each corporate bond yield 𝑑𝑦i, by a movement in z at the closest maturity and in 𝑠 for the same credit rating. The remaining component is si
The movement in the value, say 𝑊 then is
where 𝐷𝑉𝐵𝑃 is the total dollar value of a basis point for the associated risk factor.
This leads to a total risk decomposition of
A greater number of general risk factors should create less residual risk. In practice, there may not be sufficient history to measure the specific risk of individual bonds, which is why i is often assumed that all issuers within the same risk class have the same risk.
Three Approaches For Fixed Income Portfolios
- The three approaches to mapping portfolios of fixed income securities are:
- Principal mapping – One risk factor is chosen that corresponds to the average portfolio maturity. Coupon is stripped out and only principal repayment is considered at maturity.
- Duration mapping – One risk factor is chosen that corresponds to the portfolio duration. Coupons are incorporated along with the principal.
- Cash flow mapping – Several risk factor are chosen and the bond risk is decomposed into risk of each of the bond cash flows. The portfolio cash flows are grouped into maturity buckets. Cash flow mapping is the most precise method because present values of the cash flows are considered, and inter-maturity correlations are included.
Mapping Fixed Income Portfolios
| Term | Yield | Yield VaR | Modified Duration | Returns VaR |
|---|---|---|---|---|
| 1 | 5.830% | 0.00497401 | 0.944911651 | 0.470% |
| 2 | 5.710% | 0.005216789 | 1.891968593 | 0.987% |
| 3 | 5.810% | 0.005234068 | 2.835270768 | 1.484% |
| 4 | 5.890% | 0.00521773 | 3.777504958 | 1.971% |
| 5 | 5.960% | 0.005141179 | 4.718761797 | 2.426% |
| 7 | 6.070% | 0.004836792 | 6.59941548 | 3.192% |
| 9 | 6.200% | 0.00462442 | 8.474576271 | 3.919% |
| 10 | 6.260% | 0.00451605 | 9.410878976 | 4.250% |
| Term (Year) | Cash Flows | Spot Rate | Mapping (PV) | |||
|---|---|---|---|---|---|---|
| 5 Year Bond | 1 Year Bond | Principal | Duration | Cash Flow | ||
| 1 | $6 | $104 | 4.000% | 0 | 0 | $105.77 |
| 2 | $6 | 0 | 4.618% | 0 | 0 | $5.48 |
| 2.733 | — | — | — | — | $200 | — |
| 3 | $6 | 0 | 5.192% | $200 | 0 | $5.15 |
| 4 | $6 | 0 | 5.716% | 0 | 0 | $4.80 |
| 5 | $106 | 0 | 6.112% | 0 | 0 | $78.79 |
| Total | — | — | $200 | $200 | $200 | |
Mapping Fixed Income Portfolios-CF Mapping
| Term (Year) | PV Cash Flows | Individual VaR | Correlation Matrix R | Component VaR | |||||
|---|---|---|---|---|---|---|---|---|---|
| x | x × V | 1Y | 2Y | 3Y | 4Y | 5Y | (xΔVaR) | ||
| 1 | $105.77 | 0.4966 | 1 | – | – | – | – | $0.45 | |
| 2 | $5.48 | 0.0540 | 0.897 | 1 | – | – | – | $0.05 | |
| 3 | $5.15 | 0.0765 | 0.886 | 0.991 | 1 | – | – | $0.08 | |
| 4 | $4.80 | 0.0947 | 0.866 | 0.976 | 0.994 | 1 | – | $0.09 | |
| 5 | $78.79 | 1.9115 | 0.855 | 0.966 | 0.988 | 0.998 | 1 | $1.90 | |
| Total | $200.00 | 2.6335 | |||||||
| Undiversified VaR | $2.63 | ||||||||
| Diversified VaR | $2.57 | ||||||||
Three Approaches For Fixed Income Portfolios
- Cash Flow mapping VaR is less than the duration VaR because of two reasons :
- First, risk measures are not perfectly linear with maturity
- Second, correlations are below unity, which reduces risk even further.
- Out of the total $130,000 difference ($2.70 million – $2.57 million) in these measures, $70,000 ($2.70-$2.63 million) is due to differences in yield volatility, and the remaining $60,000 is due to imperfect correlations.
Stress Testing
COMPUTING VAR FROM CHANGE IN PRICE OF ZEROS
| Term (Year) | Cash Flows | Spot Rate | Old Discount Factor | PV Of CF | Risk (%) | New Discount Factor | New PV of CF |
|---|---|---|---|---|---|---|---|
| 1 | $110 | 4.000% | 0.96154 | $105.769 | 0.470% | 0.95702 | $105.272 |
| 2 | $6 | 4.618% | 0.91367 | $5.482 | 0.987% | 0.90465 | $5.428 |
| 3 | $6 | 5.192% | 0.85912 | $5.155 | 1.484% | 0.84637 | $5.078 |
| 4 | $6 | 5.716% | 0.80064 | $4.804 | 1.971% | 0.78486 | $4.709 |
| 5 | $106 | 6.112% | 0.74332 | $78.792 | 2.426% | 0.72529 | $76.881 |
| Total | $200.00 | $197.368 | |||||
| Loss | $2.634 | ||||||
Benchmarking
| Vertex | Risk (%) | Position: Index ($) | Position: Portfolio | ||||
|---|---|---|---|---|---|---|---|
| 1 ($) | 2 ($) | 3 ($) | 4 ($) | 5 ($) | |||
| ≤1m | 0.022 | 1.05 | 0.0 | 0.0 | 0.0 | 0.0 | 84.8 |
| 3m | 0.065 | 1.35 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 6m | 0.163 | 2.49 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 1Y | 0.470 | 13.96 | 0.0 | 0.0 | 59.8 | 0.0 | 0.0 |
| 2Y | 0.987 | 24.83 | 0.0 | 62.6 | 0.0 | 0.0 | 0.0 |
| 3Y | 1.484 | 15.40 | 0.0 | 59.5 | 0.0 | 0.0 | 0.0 |
| 4Y | 1.971 | 11.57 | 38.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 5Y | 2.426 | 7.62 | 62.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 7Y | 3.192 | 6.43 | 0.0 | 40.5 | 0.0 | 0.0 | 0.0 |
| 9Y | 3.913 | 4.51 | 0.0 | 37.4 | 0.0 | 0.0 | 0.0 |
| 10Y | 4.250 | 3.34 | 0.0 | 0.0 | 40.2 | 0.0 | 0.0 |
| 15Y | 6.234 | 3.00 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 20Y | 8.146 | 3.15 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 30Y | 11.119 | 1.31 | 0.0 | 0.0 | 0.0 | 0.0 | 15.2 |
| Total | 100.00 | 100.00 | |||||
| Duration | 4.62 | ||||||
| Absolute VaR | $1.99 $2.25 $2.16 $2.04 $1.94 $1.71 | ||||||
| Tracking error VaR | $0.00 $0.43 $0.29 $0.16 $0.20 $0.81 | ||||||
This table presents the cash-flow decomposition of the J.P. Morgan U.S. bond index, which has a duration of 4.62 years. Assume that we are trying to benchmark a portfolio of $100 million. Over a monthly horizon, the VaR of the index at the 95 percent confidence level is $1.99 million. This is about equivalent to the risk of a 4-year note.
- We try to match the index with two bonds. The rightmost columns in the table display the positions of two-bond portfolios with duration matched to that of the index. Since no zero- coupon has a maturity of exactly 4.62 years, the closest portfolio consists of two positions, each in a 4- and a 5-year zero. The respective weights for this portfolio are $38 million and $62 million.
If we define the new vector of positions for this portfolio as 𝑥 and for the index as 𝑥0. The VaR of the deviation relative to the benchmark is
Mapping Forwards
- From Part 1, we know that if we assume continuous compounding, then the current value of the forward contract is
And for discrete compounding it can be written as
For currency forwards, the foreign interest rate will take the place of 𝑦 in the above equations.
Apart from that it can also be expressed as the is the present value of the difference between the current forward rate and the locked-in delivery rate, i.e.
So, if we are long a forward contract with contracted rate 𝐾, we can liquidate the contract by entering a new contract to sell at the current rate 𝐹t. This will lock in a profit of (𝐹t – 𝐾), which we need to discount to the present time to find 𝐹t .
Risk and Correlations for Forward Contract Risk Factors (monthly VaR at 95 percent level)
| Risk Factor | Price or Rate | VaR (%) | Correlations | ||
|---|---|---|---|---|---|
| EUR Spot | EUR 1Y | USD 1Y | |||
| EUR spot | $1.2877 | 0.0400 | |||
| Long EUR bill | 2.2810% | -0.0583 | |||
| Short EUR bill | 3.3304% | 0.2121 | 0.0400 | -0.0583 | 1 |
| EUR forward | $1.3009 | ||||
- This table examines the risk of a 1-year forward contract to purchase 100 million euros in exchange for $130.086 million. The first step is to find the market value of the contract using the previous equation for discrete compounding
Thus the initial value of the contract is zero. This value may change, creating market risk.
- Among the three sources of risk, the volatility of the spot contract is the highest by far, with a 4.54 percent VaR (corresponding to 1.65 standard deviations over a month for a 95 percent confidence level). This is much greater than the 0.14 percent VaR for the EUR 1-year bill or even the 0.21 percent VaR for the USD bill. Thus most of the risk of the forward contract is driven by the cash EUR position.
- But risk is also affected by correlations. The positive correlation of 0.13 between the EUR spot and bill positions indicates that when the EUR goes up in value against the dollar, the value of a 1-year EUR investment is likely to appreciate. Therefore, higher values of the EUR are associated with lower EUR interest rates. This positive correlation increases the risk of the combined position. On the other hand, the position is also short a 1-year USD bill, which is correlated with the other two legs of the transaction. The issue is, what will be the net effect on the risk of the forward contract?
Computing VaR for a EUR 100 Million Forward Contract (monthly VaR at 95 percent level)
| Position | Present-value Factor | Cash Flow (CF) | PV of Flows, \(x\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| EUR spot | — | $125.89 | $5.713 | $5.704 | |
| Long EUR bill | 0.977698 | EUR100.00 | $125.89 | $0.176 | $0.029 |
| Short EUR bill | 0.967769 | —130.09 | —125.89 | $0.267 | $0.002 |
| Undiversified VaR | — | $6.156 | — | ||
| Diversified VaR | — | — | $5.735 | ||
- A long position in a forward contract that is used to purchase foreign currency with USD, one year from now, has three components:
- Long position in foreign currency spot i.e. a long spot position in EUR, worth EUR 100 million = $130.09 million in a year, or ((𝑆𝑒–rc) = $125.89 million now
- Long position in foreign currency bill i.e. a long position in a EUR investment, also worth $125.89 million now,
- Short position in USD bill i.e. a short position in a USD investment, worth $130.09 million i a year, or (𝐾𝑒–rc) = $125.89 million now.
- Considering only the spot position, the VaR is $125.89 million times the risk of 4.538 percent, which is $5.713 million. To compute the diversified VaR, we use the risk matrix from the data in the previous table and pre- and post-multiply by the vector of positions (PV of flows column in the table). The total VaR for the forward contract is $5.735 million. This number is about the same size as that of the spot contract because exchange-rate volatility dominates the volatility of 1-year bonds.
- More generally, the same methodology can be used for long-term currency swaps, which are equivalent to portfolios of forward contracts. For instance, a 10-year contract to pay dollars and receive euros is equivalent to a series of 10 forward contracts to exchange a set amount of dollars into marks. To compute the VaR, the contract must be broken down into a currency-risk component and a string of USD and EUR fixed-income components. As before, the total VaR will be driven primarily by the currency component.
Mapping Commodity Forwards
- The valuation of forward or futures contracts on commodities is substantially more complex than for financial assets such as currencies, bonds, or stock indices. Most commodities do not make monetary payments but instead are consumed, thus creating an implied benefit. This flow of benefit, net of storage cost, is loosely called convenience yield to represent the benefit from holding the cash product. This convenience yield, however, is not tied to another financial variable, such as the foreign interest rate for currency futures. It is also highly variable, creating its own source of risk.
- Monthly VaR measures for commodity futures are very high, in contrast to currency and equity market VaRs. Thus commodities are much more volatile than typical financial assets.
- Volatilities decrease with maturity of commodity futures contracts. The effect is strongest for less storable products such as energy products and less so for base metals. And this effect is very light for precious metals, which have low storage costs and no convenience yield. For financial assets, volatilities are driven primarily by spot prices, which implies basically constant volatilities across contract maturities.
- The forward rate agreement can be decomposed into two zero coupon components. If long position in a 6 x 12 FRA is considered, the components are:
- Long position in a 6-month bill
- Short position in a 12-month bill
Computing the VaR of a $100 FRA (monthly VaR at 95 percent level)
| Position | PV of Flows \(x\) | Risk % \(V\) | Correlation Matrix \(R\) | Individual VaR, \(|x|V\) | Component VaR, \(x \Delta VaR\) |
|---|---|---|---|---|---|
| 180 days | —$97.264 | 0.1629 | 1 0.8738 |
$0.158 | $5.704 |
| 360 days | $97.264 | 0.4696 | 0.8738 1 |
$0.457 | $0.029 |
| Undiversified VaR | — | $0.615 | — | ||
| Diversified VaR | — | — | $0.327 | ||
his table provides a worked-out example. If the 360-day spot rate is 5.8125 percent and the 180-day rate is 5.6250 percent, the forward rate must be such that
The present value of the notional $100 million in 6 months is
This amount is invested for 12 months.
Mapping Forward Rate Agreements
- The previous table also displays the computation of VaR for the FRA. The VaRs of 6- and 12-month zeroes are 0.1629 and 0.4696, respectively, with a correlation of 0.8738. Applied to the principal of $97.26 million, the individual VaRs are $0.158 million and $0.457 million, which gives an undiversified VaR of $0.615 million. Fortunately, the correlation substantially lowers the FRA risk. The largest amount the position can lose over a month at the 95 percent level is $0.327 million.
Mapping Interest Rate Swap
- Interest-rate swaps are the most actively used derivatives. They create exchanges of interest- rate flows from fixed to floating or vice versa. Swaps can be decomposed into two legs, a fixed leg and a floating leg. The fixed leg can be priced as a coupon-paying bond; the floating leg is equivalent to a floating-rate note (FRN).
- To illustrate, let us compute the VaR of a $100 million 5-year interest-rate swap. We enter a dollar swap that pays 6.195 percent annually for 5 years in exchange for floating-rate payments indexed to London Interbank Offer Rate (LIBOR). Initially, we consider a situation where the floating-rate note is about to be reset. Just before the reset period, we know that the coupon will be set at the prevailing market rate. Therefore, the note carries no market risk, and its value can be mapped on cash only. Right after the reset, however, the note becomes similar to a bill with maturity equal to the next reset period.
- Interest-rate swaps can be viewed in two different ways: as (1) a combined position in a fixed- rate bond and in a floating-rate bond or (2) a portfolio of forward contracts. We first value the swap as a position in two bonds using risk VaR data from the earlier table that we used to map fixed income securities. The analysis is detailed in table given in the next slide.
Computing the VaR of a $100 million Interest Rate Swap (monthly VaR at 95 percent level)
| Term (Year) | Cash Flow | Spot Rate | PV of Net Cash Flow | Individual VaR | Component VaR | |
|---|---|---|---|---|---|---|
| Fixed | Floating | |||||
| 0 | $0 | +100 | —$100.000 | $0 | $0 | |
| 1 | —$6.195 | $0 | 5.813% | —$5.855 | $0.027 | $0.024 |
| 2 | —$6.195 | $0 | 5.929% | —$5.521 | $0.054 | $0.053 |
| 3 | —$6.195 | $0 | 6.034% | —$5.196 | $0.077 | $0.075 |
| 4 | —$6.195 | $0 | 6.130% | —$4.833 | $0.096 | $0.096 |
| 5 | —$106.195 | $0 | 6.217% | —$78.546 | $1.905 | $1.905 |
| Total | $0.000 | |||||
| Undiversified VaR | $2.160 | |||||
| Diversified VaR | $2.152 | |||||
- The second and third columns lay out the payments on both legs. Just before reset, the long position in the FRN is worth $100 million, and the market value of the swap is zero. To clarify the allocation of current values, the FRN is allocated to cash, with a zero maturity. This has no risk. The next column lists the zero-coupon swap rates for maturities going from 1 to 5 years. The fifth column reports the present value of the net cash flows, fixed minus floating. The last column presents the component VaR, which adds up to a total diversified VaR of $2.152 million. The undiversified VaR is obtained from summing all individual VaRs. As usual, the $2.160 millio value somewhat overestimates risk.
- This swap can also be viewed as the sum of five forward contracts, as shown in this table. The 1-year contract promises payment of $100 million plus the coupon of 6.195 percent; discounted at the spot rate of 5.813 percent, this yields a present value of -$100.36 million. This is in exchange for $100 million now, which has no risk.
An Interest-Rate Swap Viewed as Forward Contracts (monthly VaR at 95 percent level)
| Term (Year) | PV of Flows: Contract | VaR | ||||
|---|---|---|---|---|---|---|
| 1 | 1 × 2 | 2 × 3 | 3 × 4 | 4 × 5 | ||
| 1 | —$100.36 | $94.50 | ||||
| 2 | -$94.64 | $89.11 | ||||
| 3 | $89.08 | $83.88 | ||||
| 4 | -$83.70 | $78.82 | ||||
| 5 | —$78.55 | |||||
| VaR | $0.471 | $0.571 | $0.488 | $0.446 | $0.425 | |
| Undiversified VaR | $2.401 | |||||
| Diversified VaR | $2.152 | |||||
- The next contract is a 1 x 2 forward contract that promises to pay the principal plus the fixed coupon in 2 years, or -$106.195 million; discounted at the 2-year spot rate, this yields -$94.64 million. This is in exchange for $100 million in 1 year, which is also $94.50 million when discounted at the 1-year spot rate. And so on until the fifth contract, a 4 x 5 forward contract.
- The previous table also shows the VaR of each contract. The undiversified VaR of $2.401 million is the result of a simple summation of the five VaRs. The fully diversified VaR is
- $2.152 million, exactly the same as in the preceding table. This demonstrates the equivalence of the two approaches.
- Finally, we examine the change in risk after the first payment has just been set on the floating- rate leg. The FRN then becomes a 1-year bond initially valued at par but subject to fluctuations in rates. The only change in the pattern of cash flows in Table 4-10 is to add $100 million to the position on year 1 (from -$5.855 to $94.145). The resulting VaR then decreases from $2.152 million to $1.763 million. More generally, the swap’s VaR will converge to zero as the swap matures, dipping each time a coupon is set.
Mapping Options
- Let’s consider the mapping process for nonlinear derivatives, or options. Obviously, this nonlinearity may create problems for risk measurement systems based on the delta-normal approach, which is fundamentally linear. To simplify, consider the Black-Scholes (BS) model for European options. From Part 1, we remember that
where 𝑁(𝑑) is the cumulative normal distribution function with arguments, and
where 𝐾 is now the exercise price at which the option holder can, but is not obligated to, buy the asset.
- Delta, is particularly important for options. For a European call, this is
. This figure displays its behavior as a function of the underlying spot price and for various maturities. The figure shows that delta is not a constant, which may make linear methods inappropriate for measuring the risk of options. Delta increases with the underlying spot price. The relationship becomes more nonlinear for short-term options, for example, with an option maturity of 10 days. Linear methods approximate delta by a constant value over the risk horizon. The quality of this approximation depends on parameter values.

For instance, if the risk horizon is 1 day, the worst down move in the spot price is
leading to a worst price of $97.92. With a 90-day option, delta changes from 0.536 to 0.452 only. With such a small change, the linear effect will dominate the nonlinear effect. Thus linear approximations may be acceptable for options with long maturities when the risk horizon is short.
- In the extreme case, where the option is deep in the money, both 𝑁(𝑑1) and 𝑁(𝑑2) are equal to unity, and the option behaves exactly like a position in a forward contract. In this case, the BS model reduces to 𝑐 = 𝑆𝑒–r∗c — 𝐾𝑒–rc, which is indeed the valuation formula for a forward contract. Also note that the position on the dollar bill 𝐾𝑒–rc𝑁 𝑑2 is equivalent to
This shows that the call option is equivalent to a position of Δ in the underlying asset plus a short position of Δ𝑆 — 𝑐 in a dollar bill, that is
𝐿𝑜𝑛𝑔 𝑜𝑝𝑡𝑖𝑜𝑛 = 𝑙𝑜𝑛𝑔 Δ 𝑎𝑠𝑠𝑒𝑡 + 𝑠ℎ𝑜𝑟𝑡 Δ𝑆 — 𝑐 𝑏𝑖𝑙𝑙
For example, assume that the delta for an at-the-money call option on an asset worth $100 is Δ =
0.536. The option itself is worth $4.20. This option is equivalent to a ΔS = $53.60 position in the underlying asset financed by a loan of ΔS – c = $53.60 – $4.20 = $49.40.
- The next step in the risk measurement process is the aggregation of exposures across the portfolio. Thus all options on the same underlying risk factor are decomposed into their delta equivalents, which are summed across the portfolio. This generalizes to movements in the implied volatility, if necessary. The option portfolio would be characterized by its net vega, or Λ. This decomposition also can take into account second-order derivatives using the net gamma, or Γ. These exposures can be combined with simulations of the underlying risk factors to generate a risk distribution.





